Math 4 Wisdom. "Mathematics for Wisdom" by Andrius Kulikauskas.

Investigation: Interpret and systematize the key formulas of universal hyperbolic geometry.

Universal hyperbolic geometry

Write down all of the results of Universal Hyperbolic Geometry. Organize them and interpret them in terms of

cross-ratio
symmetric functions of variables
matrices and symmetric functions of eigenvalues
Lie theory (Lie bracket, Jacobi identity...)
Which formulas are symmetric in their variables, and which are not symmetric, and why?
Look at Wildberger's three binormal forms in chromogeometry.

Understand and interpret

Understand the dot product, especially in three dimensions. Why are u and v perpendicular iff their dot product is zero?
Understand the equations for a line and a plane. How should I understand the minus sign -nz ?
Understand how projection occurs on the plane z=1.
What does it mean to calculate the spread and quadrance as cross-ratios?
In what sense does the anharmonic ratio -1, 1/2, 2 collapse the trigonometric functions from six to three?
Understand Pythagoras's theorem.
- How does it come from the definition of the cross-ratio?
- And what is a geometric interpretation?
- Does the general Pythagoras's theorem work for an ellipse (and hyperbola) rather than just a circle?
- How does the law of cosines interpolate between linear addition a + b and quadratic addition {$a^2+b^2$}.
- Relate the motion of the line in the law of cosines to polarity.
What is the role of concurrency, colinearity in all of the formulas?
In what sense does the number i relate spread (and sin2 and trigonometric functions) and quadrance (and sinh2 and hyperbolic functions)?
Understand the relationship between the Lie bracket and dual points and lines.
{$1-q_1$}
{$q_1+q_2+q_3$}

Exercises

Formulate analogues of the deep ideas behind geometry and trigonometry, such as: A right triangle is half a rectangle. A triangle is the sum of two right triangles. Four times a right triangle is the difference of two squares.

Literature

Duality

In hyperbolic geometry, the quadrance {$q$} (between points) and spread {$S$} (between lines) are dual. Each is equal to the cross-ratio.

Quadrance Spread Duality Theorem. If {$a_1=A_1^{\perp}$} and {$a_2=A_2^{\perp}$}, then {$R(a_1,b_2;a_2,b_1)=q(a_1,a_2)=S(A_1,A_2)$}. Where {$a_1,a_2$} are points within a circle and {$b_1,b_2$} are points on their respective polars.

Foundations

In the plane, two vectors indicate the same line if the area they span is zero.

{$x_1:y_1 = x_2:y_2 \iff \frac{x_1}{y_1} = \frac{x_2}{y_2} \iff x_1y_2 - x_2y_1 = 0$}

In three-dimensional space, two vectors indicate the same line if, in each of the three planes, the area they span is zero.

{$x_1:y_1:z_1 = x_2:y_2:z_2 \iff \frac{x_1}{y_1} = \frac{x_2}{y_2} \wedge \frac{x_1}{z_1} = \frac{x_2}{z_2} \wedge \frac{y_1}{z_1} = \frac{y_2}{z_2} \iff x_1y_2 - x_2y_1 = x_1z_2 - x_2z_1 = y_1z_2 - y_2z_1 = 0$}

Given point a = [x:y:z] and line L = (l:m:n).

a is on L (L passes through a) when {$xl+my=nz$} or when {$xl+my-nz=0$} or when {$a\circ L=0$}.

a and L are dual ({$a^{\perp}=L$} and {$a=L^{\perp}$}) when [x:y:z]=[l:m:n] or (x:y:z)=(l:m:n).

a is null when it lies on its dual line, thus when {$x^2+y^2=z^2$}, (when {$a\circ a^{\perp}=0$}), and likewise, L is null when it passes through its dual point.

Points {$a_1=[x_1:y_1:z_1]$} and {$a_2=[x_2:y_2:z_2]$} are perpendicular when {$x_1x_2+y_1y_2=z_1z_2$} or when {$x_1x_2+y_1y_2-z_1z_2=0$} or when {$a_1\circ a_2=0$}. Similarly with lines.

Overview

We have laws for:

{$q_1q_2=S_1S_2$} Spread law.
{$q_1q_2q_3$} Triple quad law.
{$q_1q_2S_3=q_1S_2q_3=S_1q_2q_3$} Cross law. These terms give the square of the area of the parallelogram whose sides have quadrances {$q_1$} and {$q_2$} separated by spread {$S_3$}.
{$S_1S_2q_3=S_1q_2S_3=q_1S_2S_3$} Cross dual law.
{$S_1S_2S_3$} Triple Spread law.

The squares of the six trigonometric functions find expression as the six possible cross-ratios: q = sin2, 1-q = cos2, 1/q = csc2, 1/(1-q) = sec2, q/(1-q) = tan2, (1-q)/q = cot2. Similarly, the six hyperbolic functions express the quadrance.

Symmetric formulas

Spread law. {$\frac{S_1}{q_1}=\frac{S_2}{q_2}=\frac{S_3}{q_3}$}

Triple quad formula. If {$a_1, a_2, a_3$} collinear, then {$(q_1+q_2+q_3)^2=2(q_1^2+q_2^2+q_3^2)+4q_1q_2q_3$}, which is {$p_1^2=2p_2+4e_3$}.

Triple spread formula. If {$A_1, A_2, A_3$} concurrent, then {$(S_1+S_2+S_3)^2=2(S_1^2+S_2^2+S_3^2)+4S_1S_2S_3$}, which is {$p_1^2=2p_2+4e_3$}.

These formula can be rewritten: {$q_1q_2q_3=(2(q_1^2+q_2^2+q_3^2)-(q_1+q_2+q_3)^2)/4$}, in other words, {$e_3=(2p_2-p_1^2)/4$}

Nonsymmetric formulas

Pythagoras's theorem. If {$a_1a_3\perp a_2a_3$} then {$q_3=q_1+q_2-q_1q_2$}. More elegantly: {$(1-q_3)=(1-q_1)(1-q_2)$}.

Pythagoras's dual theorem. If {$A_1A_3\perp A_2A_3$} then {$S_3=S_1+S_2-S_1S_2$}. More elegantly: {$(1-S_3)=(1-S_1)(1-S_2)$}. Alternatively, {$|\textrm{cos}\,\theta_3|=|\textrm{cos}\,\theta_1||\textrm{cos}\,\theta_2|$}.

{$(q_1q_2S_3-(q_1+q_2+q_3)+2)^2=4(1-q_1)(1-q_2)(1-q_3)$}

Cross law. {$(q_1q_2S_3-(q_1+q_2+q_3)+2)^2=4(1-q_1)(1-q_2)(1-q_3)$}

{$q_1q_2S_3=q_1S_2q_3=S_1q_2q_3= q_1+q_2+q_3 -2 \pm 2\sqrt{(1-q_1)(1-q_2)(1-q_3)}$}

Cross dual law. {$(S_1S_2q_3-(S_1+S_2+S_3)+2)^2=4(1-S_1)(1-S_2)(1-S_3)$}

{$S_1S_2q_3=S_1q_2S_3=q_1S_2S_3= S_1+S_2+S_3 -2 \pm 2\sqrt{(1-S_1)(1-S_2)(1-S_3)}$}

Note that {$\sqrt{1-S_i}=|\textrm{cos}\,\theta_i|$}

Theorems

The altitudes of a triangle meet at a point (the orthocenter).

Affine geometry

Lines are parallel:

All (n 2) slopes the same.
Collinearity: Triple quad formula

Affine combinations

Vector is the relation between parallel lines.

Projective geometry

Space is divided into "infinity" and "finity".

Conformal geometry

Lines are perpendicular: Pythagorean theorem, inner product

Quadratic interpolations: law of cosines A2 - 2ABcos(theta) + B2 = C2 interpolation of (A-B)2 = C2, A2 + B2 = C2, (A+B)2 = C2. Consider triangles more generally. Or consider the function: A2 + B2 - C2 which is 0 when A and B are perpendicular, and nonzero 2ABcos(theta) otherwise.

Inner product defines the equation of a line and whether points lie on it. (a1, a2, a3)(x1, x2, 1) = 0.

Symplectic geometry

Oriented area, volume given by determinant.

Quadrea: 4 x determinant squared. Determinant is positive three cycle and negative three cycle.
Quadrea: Archimedes function (and when zero, we have collinearity by the Triple quad formula.
Quadrea: Can repeatedly factor Archimedes function as a2-b2 = (a+b)(a-b) to get Heron's law for the area in terms of the lengths of the edges.

What if we interpret the line as ax + by + cz = 0 where z=1 ?

Why is the s1s2s3 term of the third power and not the second power?

Notes

The circle maps every point to a line and vice versa.
Quadrance (distance squared) is more correct than distance because quadrance makes positive distance and negative distance equivalent. Spread (absolute value of the sine of angle) is more correct than angle because the value of the spread is the same for all angles at an intersection, which is to say, for both theta and pi minus theta. In this way, quadrance and spread eliminate false distinctions and the problems they cause.
How Chromogeometry transcends Klein's Erlangen Program for Planar Geometries| N J Wildberger
Try to express projective geometry (or universal hyperbolic geometry) in terms of matrices and thus symmetric functions. What then is algebraic geometry and how do polynomials get involved? What is analytic geometry and in what sense does it go beyond matrices? How do all of these hit up against the limits of matrices and the amount of symmetry in its internal folding?

N J Wildberger. Chromogeometry

Symmetric bilinear forms given vectors {$A_1=[x_1,y_1]$} and {$A_2=[x_2,y_2]$}

parallel if {$x_1y_2-x_2y_1=0$}
blue (Euclidean) {$[x_1,y_1]\cdot_{b}[x_2,y_2]=x_1x_2+y_1y_2$}
red (relativistic) {$[x_1,y_1]\cdot_{r}[x_2,y_2]=x_1x_2-y_1y_2$}
green (relativistic) {$[x_1,y_1]\cdot_{b}[x_2,y_2]=x_1y_2+x_2y_1$}

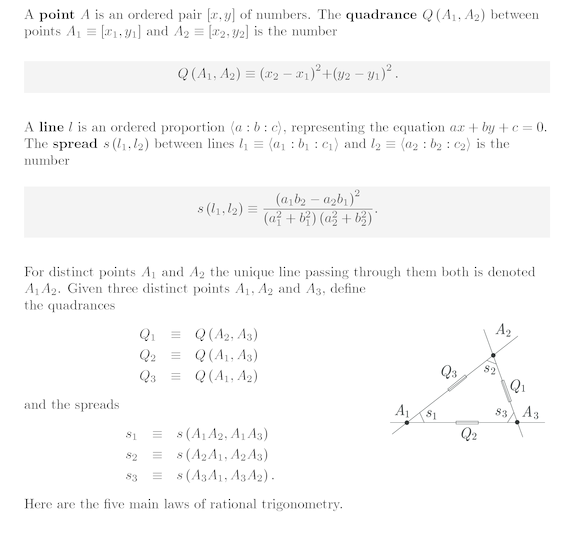
Definitions

For any two points {$A_1 ≡ [x_1, y_1]$} and {$A_2 ≡ [x_2, y_2]$} there is a unique line {$l ≡ A_1A_2$} which passes through them both.
The quadrance between the points {$A_1$} and {$A_2$} is the number {$Q (A_1, A_2) ≡ (A_2 − A_1) · (A_2 − A_1)$}. It is the distance squared.
The spread between the non-null lines {$A_1A_2$} and {$B_1B_2$} is the number

{$$s(A_1A_2, B_1B_2) ≡ 1 − \frac{((A_2 − A_1) · (B_2 − B_1))^2}{Q(A_1, A_2) Q(B_1, B_2)}$$}. This is {$\textrm{sin}^2\theta$}. It is independent of the choice of points lying on the two lines. Two non-null lines are perpendicular precisely when the spread between them is 1.

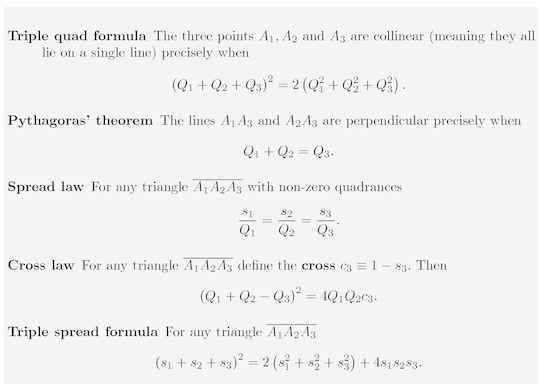
Five main laws of planar rational trigonometry

Cross Law. {$(Q_1 + Q_2 − Q_3)^2 = 4Q_1Q_2 (1 − s_3)$} This is the square of the law of cosines: {$c^{2}=a^{2}+b^{2}-2ab\cos \gamma $}. It relates the angles and the lengths of sides of a triangle. Two special cases:
- Triple Quad Formula. The points {$A_1$}, {$A_2$} and {$A_3$} are collinear precisely when the quadrances {$Q_1 ≡ Q(A_2, A_3)$}, {$Q_2 ≡ Q(A_1, A_3)$} and {$Q_3 ≡ Q(A_1, A_2)$} satisfy {$(Q_1 + Q_2 + Q_3)^2 = 2 (Q_1^2 + Q_2^2 + Q_3^2)$}.
- Pythagoras's Theorem. For {$A_1$}, {$A_2$} and {$A_3$} three distinct points, {$A_1A_3$} is perpendicular to {$A_2A_3$} precisely when the quadrances {$Q_1 ≡ Q (A_2, A_3)$}, {$Q_2 ≡ Q(A_1, A_3)$} and {$Q_3 ≡ Q(A_1, A_2)$} satisfy {$Q_1 + Q_2 = Q_3$}.
Spread Law. For a triangle, {$s_1/Q_1=s_2/Q_2=s_3/Q_3$}. This is the law of sines, and relates angles and lengths of sides for a triangle.
Triple spread formula. {$(s_1 + s_2 + s_3)^2 = 2 (s_1^2 + s_2^2 + s_3^2) + 4s_1s_2s_3$}. Relates the angles of a triangle and generalizes the formula for the sum of the angles.

Videos

Norman Wildberger
- Triangle geometry
- Universal Hyperbolic Geometry
  - UnivHypGeom4: First steps in hyperbolic geometry: fundamental results
- Algebraic calculus one
Affine and projective universal geometry by Norman Wildberger
One dimensional metrical geometry
Chromogeometry