Introduction E9F5FC

Questions FFFFC0

• View
• Edit
• History
• Print

Bott Periodicity for Octonion Maniacs

Investigating Bott periodicity

L4 decouples the three minds

They appear independently

The others are beyond the mirror

Time and Space as Representations of Decision-Making

John Baez. The Octonions.

The Yoneda Embedding Expresses Whether, What, How, Why

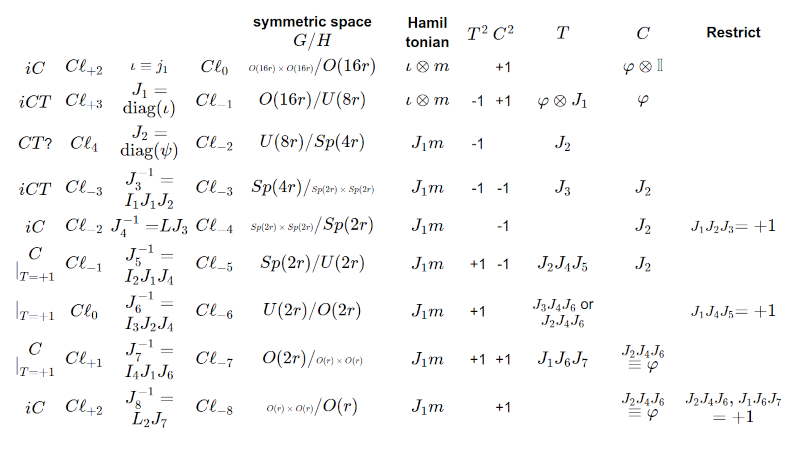
Sets of Mutually Anticommuting Linear Complex Structures

Goal: Describe mutually anticommuting linear complex structures {$J_1, J_2, J_3, J_4, J_5, J_6, J_7,\cdots$}

Orthogonal matrices {$J_\alpha$} such that {$J_\alpha^2=-I$} and {$J_\alpha J_\beta =-J_\beta J_\alpha$}.

Note that if {$J_\alpha,J_\beta,J_\gamma$} are distinct, then {$(J_\alpha J_\beta)^2=-I$} but {$(J_\alpha J_\beta J_\gamma)^2=I$}.

Notation for {$2\times 2$} blocks thought of as linear (complex numbers) or antilinear

{$I_2= \begin{pmatrix} 1 & 0 \\ 0 & 1 \\ \end{pmatrix}, i_2= \begin{pmatrix} 0 & -1 \\ 1 & 0 \\ \end{pmatrix}, r_2= \begin{pmatrix} 1 & 0 \\ 0 & -1 \\ \end{pmatrix}, i_2r_2= \begin{pmatrix} 0 & 1 \\ 1 & 0 \\ \end{pmatrix}$}

Notation for {$4\times 4$} blocks thought of as quaternions

{$i_4= \begin{pmatrix} 0 & -1 & 0 & 0 \\ 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & -1 \\ 0 & 0 & 1 & 0 \\ \end{pmatrix}, j_4= \begin{pmatrix} 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & -1 \\ -1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ \end{pmatrix}, k_4= \begin{pmatrix} 0 & 0 & 0 & 1 \\ 0 & 0 & 1 & 0 \\ 0 & -1 & 0 & 0 \\ -1 & 0 & 0 & 0 \\ \end{pmatrix}$}

{$i_4j_4=k_4,\;\; j_4k_4=i_4,\;\; k_4i_4=j_4,\;\;i^2=j^2=k^2=-1,\;\;,j_4i_4=-k_4,\;\; k_4j_4=-i_4,\;\; i_4k_4=-j_4$}

---

Michael Stone, Ching-Kai Chiu, Abhishek Roy. Symmetries, Dimensions, and Topological Insulators: the mechanism behind the face of the Bott clock.

They provide a recipe for constructing mutually anticommuting linear complex structures {$J_1, J_2, J_3, J_4, J_5, J_6, J_7,\cdots$}.

{$J_1=\textrm{diag}[i_2]=\textrm{diag}[i_4]$}

{$J_2=\textrm{diag}[j_4]$}

Suppose {$J_3$} exists. Let {$K=J_1J_2J_3$}. Then {$K^2=I$} and consequently, {$K$} satisfies {$x^2-1$}, thus posseses quaternionic eigenspaces {$V_{\pm}$} where it takes values {$\pm 1$} accordingly. {$Kv_+=v_+$} for {$v_+\in V_+$} and {$Kv_-=-v_-$} for {$v_-\in V_-$}. {$K$} can be used to define {$J_3=J_2^{-1}J_1^{-1}K$}.

To define {$J_4$} we must suppose {$V_+$} and {$V_-$} have the same dimension. Suppose {$J_4$} exists. Let {$L=J_3J_4$}. Then {$LK=-KL, L^2=-I, LJ_1=J_1L, LJ_2=J_2L$}. {$L$} preserves the quaternionic structure and is a quaternionic isometry from {$V_+$} to {$V_-$}. {$L$} can be used to define {$J_4=J_3^{-1}L$}.

Suppose {$J_5$} exists. Let {$M=J_1J_4J_5$}. Then {$M^2=I$} and {$M$} commutes with {$K$} and {$J_1$}. Thus {$M$} acts within an eigenspace of {$K$} (say {$V_+$}) and divides it into two mutually orthogonal eigenspaces {$W_\pm$} with {$W_-=J_2W_+$}. {$M$} can be used to define {$J_5=J_4^{-1}J_1^{-1}M$}.

Suppose {$J_6$} exists. Let {$N=J_2J_4J_6$}. Then {$N^2=I$} and {$N$} commutes with {$K$} and {$M$}. Thus {$N$} acts within an eigenspace of {$M$} (say {$W_+$}) and divides it into two mutually orthogonal eigenspaces {$X_\pm$} with {$X_-=J_1W_+$}. {$N$} can be used to define {$J_6=J_4^{-1}J_2^{-1}N$}.

Suppose {$J_7$} exists. Let {$P=J_1J_6J_7$}. Then {$P^2=I$} and {$P$} commutes with {$K$}, {$M$} and {$N$}. Thus {$P$} acts within an eigenspace of {$X$} (say {$X_+$}) and divides it into two mutually orthogonal eigenspaces {$Y_\pm$}. {$P$} can be used to define {$J_7=J_6^{-1}J_1^{-1}P$}.

To define {$J_8$} we must suppose {$Y_+$} and {$Y_-$} have the same dimension. Suppose {$J_8$} exists. Let {$Q=J_7J_8$}. Then {$Q^2=-I$} and {$Q$} commutes with {$K$}, {$M$} and {$N$} but anticommutes with {$P$}. Thus {$Q$} is an isometry mapping {$Y_+\leftrightarrow Y_-$}. {$Q$} can be used to define {$J_8=J_7^{-1}Q$}.

---

Notation

{$R_2= \begin{pmatrix} 1 & \\ & -1 \end{pmatrix}, R_4= \begin{pmatrix} 1 & & & \\ & 1 & & \\ & & -1 & \\ & & & -1 \\ \end{pmatrix}, R_8=\begin{pmatrix} 1 & & & & & & & \\ & 1 & & & & & & \\ & & 1 & & & & & \\ & & & 1 & & & & \\ & & & & -1 & & & \\ & & & & & -1 & & \\ & & & & & & -1 & \\ & & & & & & & -1 \\ \end{pmatrix}$}

The reflections {$R_2, R_4, R_8$} determine eigenspaces {$V_{+1}$} and {$V_{-1}$}.

A shift in perspective is given by an isometry between eigenspaces {$V_{+1}$} and {$V_{-1}$}.

{$L_2= \begin{pmatrix} & -1 \\ 1 & \end{pmatrix}, L_4= \begin{pmatrix} & & -1 & \\ & & & -1 \\ 1 & & & \\ & 1 & & \\ \end{pmatrix}, L_8=\begin{pmatrix} & & & & 1 & & & \\ & & & & & 1 & & \\ & & & & & & 1 & \\ & & & & & & & 1 \\ -1 & & & & & & & \\ & -1 & & & & & & \\ & & -1 & & & & & \\ & & & -1 & & & & \\ \end{pmatrix}$}

Note that

{$\begin{pmatrix} & -1 & & \\ 1 & & & \\ & & & -1 \\ & & 1 & \\ \end{pmatrix} \begin{pmatrix} & & 1 & \\ & & & 1 \\ -1 & & & \\ & -1 & & \\ \end{pmatrix} = \begin{pmatrix} & & & -1 \\ & & 1 & \\ & 1 & & \\ -1 & & & \\ \end{pmatrix}$}

{$\begin{pmatrix} 1 & & & \\ & -1 & & \\ & & 1 & \\ & & & -1 \\ \end{pmatrix} \begin{pmatrix} & & 1 & \\ & & & 1 \\ -1 & & & \\ & -1 & & \\ \end{pmatrix} = \begin{pmatrix} & & 1 & \\ & & & -1 \\ -1 & & & \\ & 1 & & \\ \end{pmatrix} = j = J_2$}

{$\begin{pmatrix} & 1 & & \\ 1 & & & \\ & & & 1 \\ & & 1 & \\ \end{pmatrix} \begin{pmatrix} & & 1 & \\ & & & 1 \\ -1 & & & \\ & -1 & & \\ \end{pmatrix} = \begin{pmatrix} & & & 1 \\ & & 1 & \\ & -1 & & \\ -1 & & & \\ \end{pmatrix} = k$}

Thus the {$2\times 2$} matrices {$M_l$} are mapped by {$L_4$} to an isometry that involves multiplying by {$M_l$} in one direction and {$-M_l$} in the opposite direction. -

---

{$J_1$}

{$J_2$}

{$J_3=D_{----++++}J_1J_2$}

{$J_4=HJ_1J_2$}

{$J_5=L_4D_{--++--++}J_2$}

{$J_6=L_4D_{-+-+-+-+}J_1$}

{$J_7=LD_{r_2-r_2-r_2r_2r_2-r_2-r_2r_2}$}

---

{$J_1$}

{$J_2$}

{$J_3=J_1J_2\begin{pmatrix} -1 & & & & & & & \\ & -1 & & & & & & \\ & & -1 & & & & & \\ & & & -1 & & & & \\ & & & & 1 & & & \\ & & & & & 1 & & \\ & & & & & & 1 & \\ & & & & & & & 1 \\ \end{pmatrix}$}

{$J_4=LJ_1J_2\begin{pmatrix} -1 & & & & & & & \\ & -1 & & & & & & \\ & & -1 & & & & & \\ & & & -1 & & & & \\ & & & & 1 & & & \\ & & & & & 1 & & \\ & & & & & & 1 & \\ & & & & & & & 1 \\ \end{pmatrix}$}

{$J_5=LJ_2\begin{pmatrix} -1 & & & & & & & \\ & -1 & & & & & & \\ & & 1 & & & & & \\ & & & 1 & & & & \\ & & & & -1 & & & \\ & & & & & -1 & & \\ & & & & & & 1 & \\ & & & & & & & 1 \\ \end{pmatrix}$}

{$J_6=LJ_1\begin{pmatrix} -1 & & & & & & & \\ & 1 & & & & & & \\ & & -1 & & & & & \\ & & & 1 & & & & \\ & & & & -1 & & & \\ & & & & & 1 & & \\ & & & & & & -1 & \\ & & & & & & & 1 \\ \end{pmatrix}$}

{$J_7=L\;\textrm{diag} \begin{bmatrix} -1 & 1 & 1 & -1 & 1 & -1 & -1 & 1 & -1 & 1 & 1 & -1 & 1 & -1 & -1 & 1 \\ \end{bmatrix}$}

xxxx

{$J_8=J_7^{-1}Q = \begin{pmatrix} & & & & ir_2 & & & \\ & & & & & -ir_2 & & \\ & & & & & & -ir_2 & \\ & & & & & & & ir_2 \\ -ir_2 & & & & & & & \\ & ir_2 & & & & & & \\ & & ir_2 & & & & & \\ & & & -ir_2 & & & & \\ \end{pmatrix}$}

---

Notation

{$\omega =\begin{pmatrix} 0 & -I_4 \\ I_4 & 0 \\ \end{pmatrix}$}

{$KL=-\omega =\begin{pmatrix} 0 & I_4 \\ -I_4 & 0 \\ \end{pmatrix}$}

---

{$J_1$}

{$J_2$}

{$J_3=J_2^{-1}J_1^{-1}K=J_2J_1K$}

{$J_4=J_3^{-1}L=K^{-1}J_1J_2L=KJ_1J_2L=(J_1J_2J_3)J_1J_2L=J_1J_2(J_1J_2J_3)L=J_1J_2KL=-J_1J_2\omega=J_2J_1\omega=J_1J_2\begin{pmatrix} 0 & 0 & I_2 & 0 \\ 0 & 0 & 0 & I_2 \\ -I_2 & 0 & 0 & 0 \\ 0 & -I_2 & 0 & 0 \\ \end{pmatrix}$}

{$J_5=J_4^{-1}J_1^{-1}M=\omega^{-1}J_1^{-1}J_2^{-1}J_1^{-1}M = \omega^{-1}J_2^{-1}M = J_2^{-1}\omega^{-1}M = J_2\begin{pmatrix} 0 & 0 & I_2 & 0 \\ 0 & 0 & 0 & -I_2 \\ I_2 & 0 & 0 & 0 \\ 0 & -I_2 & 0 & 0 \\ \end{pmatrix}$}

{$J_6=J_4^{-1}J_2^{-1}N=\omega^{-1}J_1^{-1}J_2^{-1}J_2^{-1}N=\omega^{-1}J_1^{-1}N=J_1^{-1}\omega^{-1}N=J_1\begin{pmatrix} 0 & 0 & r_2 & 0 \\ 0 & 0 & 0 & r_2 \\ r_2 & 0 & 0 & 0 \\ 0 & r_2 & 0 & 0 \\ \end{pmatrix}$}

{$J_7=J_6^{-1}J_1^{-1}P =N^{-1}\omega J_1 J_1^{-1}P=N^{-1}\omega P = \begin{pmatrix} & & & & r_2 & & & \\ & & & & & -r_2 & & \\ & & & & & & -r_2 & \\ & & & & & & & r_2 \\ -r_2 & & & & & & & \\ & r_2 & & & & & & \\ & & r_2 & & & & & \\ & & & -r_2 & & & & \\ \end{pmatrix}$}

{$J_8=J_7^{-1}Q = \begin{pmatrix} & & & & ir_2 & & & \\ & & & & & -ir_2 & & \\ & & & & & & -ir_2 & \\ & & & & & & & ir_2 \\ -ir_2 & & & & & & & \\ & ir_2 & & & & & & \\ & & ir_2 & & & & & \\ & & & -ir_2 & & & & \\ \end{pmatrix}$}

---

A concrete set of anticommuting linear complex structures {$J_1, J_2, J_3, J_4, J_5, J_6, J_7,\cdots$}

{$J_1=\textrm{diag}[i_2]=\textrm{diag}[i_4]$}

{$J_2=\textrm{diag}[j_4]$}

{$K_8=\begin{pmatrix} I_4 & 0 \\ 0 & -I_4 \\ \end{pmatrix}$}

{$m_{3_8}= \begin{pmatrix} -k_4 & 0 \\ 0 & k_4 \\ \end{pmatrix}$}

{$J_3=\textrm{diag}[m_{3_8}]$}

{$L=\begin{pmatrix} 0 & I_4 \\ -I_4 & 0 \\ \end{pmatrix}$}

{$m_{4_8}= \begin{pmatrix} 0 & k_4 \\ k_4 & 0 \\ \end{pmatrix}$}

{$J_4=\textrm{diag}[m_{4_8}]$}

{$M_4=\begin{pmatrix} I_2 & 0 \\ 0 & -I_2 \\ \end{pmatrix}$}

{$M_8=\begin{pmatrix} -M_4 & 0 \\ 0 & M_4 \\ \end{pmatrix} = \begin{pmatrix} -I_2 & 0 & 0 & 0 \\ 0 & I_2 & 0 & 0 \\ 0 & 0 & I_2 & 0 \\ 0 & 0 & 0 & -I_2 \\ \end{pmatrix}$}

{$m_{5_8}= \begin{pmatrix} 0 & 0 & 0 & -r_2 \\ 0 & 0 & -r_2 & 0 \\ 0 & r_2 & 0 & 0 \\ r_2 & 0 & 0 & 0 \\ \end{pmatrix} = \begin{pmatrix} & & & & & & -1 & \\ & & & & & & & 1 \\ & & & & -1 & & & \\ & & & & & 1 & & \\ & & 1 & & & & & \\ & & & -1 & & & & \\ 1 & & & & & & & \\ & -1 & & & & & & \\ \end{pmatrix}$}

{$J_5=\textrm{diag}[m_{5_8}]$}

{$N_4=\begin{pmatrix} r_2 & 0 \\ 0 & r_2 \\ \end{pmatrix}$}

{$N_8=\begin{pmatrix} N_4 & 0 \\ 0 & -N_4 \\ \end{pmatrix} = \begin{pmatrix} r_2 & 0 & 0 & 0 \\ 0 & r_2 & 0 & 0 \\ 0 & 0 & -r_2 & 0 \\ 0 & 0 & 0 & -r_2 \\ \end{pmatrix} = \begin{pmatrix} 1 & & & & & & & \\ & -1 & & & & & & \\ & & 1 & & & & & \\ & & & -1 & & & & \\ & & & & -1 & & & \\ & & & & & 1 & & \\ & & & & & & -1 & \\ & & & & & & & 1 \\ \end{pmatrix}$}

{$m_{6_8}= \begin{pmatrix} 0 & 0 & i_2r_2 & 0 \\ 0 & 0 & 0 & i_2r_2 \\ -i_2r_2 & 0 & 0 & 0 \\ 0 & -i_2r_2 & 0 & 0 \\ \end{pmatrix} = \begin{pmatrix} & & & & & 1 & & \\ & & & & 1 & & & \\ & & & & & & & 1 \\ & & & & & & 1 & \\ & -1 & & & & & & \\ -1 & & & & & & & \\ & & & -1 & & & & \\ & & -1 & & & & & \\ \end{pmatrix}$}

{$J_6=\textrm{diag}[m_{6_8}]$}

{$r_4=\begin{pmatrix} r_2 & 0 \\ 0 & r_2 \\ \end{pmatrix}$}

{$P_{8*}= \begin{pmatrix} r_4 & 0 & 0 & 0 \\ 0 & -r_4 & 0 & 0 \\ 0 & 0 & r_4 & 0 \\ 0 & 0 & 0 & -r_4 \\ \end{pmatrix} = \begin{pmatrix} 1 & & & & & & & & & & & & & & & \\ & -1 & & & & & & & & & & & & & & \\ & & 1 & & & & & & & & & & & & & \\ & & & -1 & & & & & & & & & & & & \\ & & & & -1 & & & & & & & & & & & \\ & & & & & 1 & & & & & & & & & & \\ & & & & & & -1 & & & & & & & & & \\ & & & & & & & 1 & & & & & & & & \\ & & & & & & & & 1 & & & & & & & \\ & & & & & & & & & -1 & & & & & & \\ & & & & & & & & & & 1 & & & & & \\ & & & & & & & & & & & -1 & & & & \\ & & & & & & & & & & & & -1 & & & \\ & & & & & & & & & & & & & 1 & & \\ & & & & & & & & & & & & & & -1 & \\ & & & & & & & & & & & & & & & 1 \\ \end{pmatrix}$}

{$s_4=\begin{pmatrix} r_2 & 0 \\ 0 & -r_2 \\ \end{pmatrix}$}

{$s_8=\begin{pmatrix} s_4 & 0 \\ 0 & -s_4 \\ \end{pmatrix}$}

{$J_7=\begin{pmatrix} 0 & s_8 \\ -s_8 & 0 \\ \end{pmatrix} = \begin{pmatrix} & & & & & & & & 1 & & & & & & & \\ & & & & & & & & & -1 & & & & & & \\ & & & & & & & & & & -1 & & & & & \\ & & & & & & & & & & & 1 & & & & \\ & & & & & & & & & & & & -1 & & & \\ & & & & & & & & & & & & & 1 & & \\ & & & & & & & & & & & & & & 1 & \\ & & & & & & & & & & & & & & & -1 \\ -1 & & & & & & & & & & & & & & & \\ & 1 & & & & & & & & & & & & & & \\ & & 1 & & & & & & & & & & & & & \\ & & & -1 & & & & & & & & & & & & \\ & & & & 1 & & & & & & & & & & & \\ & & & & & -1 & & & & & & & & & & \\ & & & & & & -1 & & & & & & & & & \\ & & & & & & & 1 & & & & & & & & \\ \end{pmatrix}$}

{$Q_{8*}= \begin{pmatrix} J_{1_4} & 0 & 0 & 0 \\ 0 & J_{1_4} & 0 & 0 \\ 0 & 0 & J_{1_4} & 0 \\ 0 & 0 & 0 & J_{1_4} \\ \end{pmatrix} = \begin{pmatrix} & -1 & & & & & & & & & & & & & & \\ 1 & & & & & & & & & & & & & & & \\ & & & -1 & & & & & & & & & & & & \\ & & 1 & & & & & & & & & & & & & \\ & & & & & -1 & & & & & & & & & & \\ & & & & 1 & & & & & & & & & & & \\ & & & & & & & -1 & & & & & & & & \\ & & & & & & 1 & & & & & & & & & \\ & & & & & & & & & -1 & & & & & & \\ & & & & & & & & 1 & & & & & & & \\ & & & & & & & & & & & -1 & & & & \\ & & & & & & & & & & 1 & & & & & \\ & & & & & & & & & & & & & -1 & & \\ & & & & & & & & & & & & 1 & & & \\ & & & & & & & & & & & & & & & -1 \\ & & & & & & & & & & & & & & 1 & \\ \end{pmatrix}$}

{$c_4= \begin{pmatrix} 0 & 1 & 0 & 0 \\ 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & -1 \\ 0 & 0 & -1 & 0 \\ \end{pmatrix}$}

{$J_8= \begin{pmatrix} 0 & 0 & c_4 & 0 \\ 0 & 0 & 0 & -c_4 \\ -c_4 & 0 & 0 & 0 \\ 0 & c_4 & 0 & 0 \\ \end{pmatrix} = \begin{pmatrix} & & & & & & & & & 1 & & & & & & \\ & & & & & & & & 1 & & & & & & & \\ & & & & & & & & & & & -1 & & & & \\ & & & & & & & & & & -1 & & & & & \\ & & & & & & & & & & & & & -1 & & \\ & & & & & & & & & & & & -1 & & & \\ & & & & & & & & & & & & & & & 1 \\ & & & & & & & & & & & & & & 1 & \\ & -1 & & & & & & & & & & & & & & \\ -1 & & & & & & & & & & & & & & & \\ & & & 1 & & & & & & & & & & & & \\ & & 1 & & & & & & & & & & & & & \\ & & & & & 1 & & & & & & & & & & \\ & & & & 1 & & & & & & & & & & & \\ & & & & & & & -1 & & & & & & & & \\ & & & & & & -1 & & & & & & & & & \\ \end{pmatrix}$}

---

{$\mathbb{C}$}

{$I=1$}	{$J_1=i$}
{$\begin{pmatrix} 1 & \\ & 1 \\ \end{pmatrix}$}	{$\begin{pmatrix} & -1 \\ 1 & \\ \end{pmatrix}$}

{$\mathbb{H}$}

{$I=1$}	{$J_1=i$}	{$J_2=j$}	{$J_1J_2=ij$}
{$\begin{pmatrix} 1 & & & \\ & 1 & & \\ & & 1 & \\ & & & 1 \\ \end{pmatrix}$}	{$\begin{pmatrix} & -1 & & \\ 1 & & & \\ & & & -1 \\ & & 1 & \\ \end{pmatrix}$}	{$\begin{pmatrix} & & 1 & \\ & & & -1 \\-1 & & & \\ & 1 & & \\ \end{pmatrix}$}	{$\begin{pmatrix} & & & 1 \\ & & 1 & \\ & -1 & & \\ -1 & & & \\ \end{pmatrix}$}

{$\mathbb{H}\oplus\mathbb{H}$}

{$\begin{pmatrix} 1 & & & & & & & \\ & 1 & & & & & & \\ & & 1 & & & & & \\ & & & 1 & & & & \\ & & & & 1 & & & \\ & & & & & 1 & & \\ & & & & & & 1 & \\ & & & & & & & 1 \\ \end{pmatrix}$}	{$\begin{pmatrix} & -1 & & & & & & \\ 1 & & & & & & & \\ & & & -1 & & & & \\ & & 1 & & & & & \\ & & & & & -1 & & \\ & & & & 1 & & & \\ & & & & & & & -1 \\ & & & & & & 1 & \\ \end{pmatrix}$}	{$\begin{pmatrix} & & 1 & & & & & \\ & & & -1 & & & & \\ -1 & & & & & & & \\ & 1 & & & & & & \\ & & & & & & 1 & \\ & & & & & & & -1 \\ & & & & -1 & & & \\ & & & & & 1 & & \\ \end{pmatrix}$}	{$\begin{pmatrix} & & & -1 & & & & \\ & & -1 & & & & & \\ & 1 & & & & & & \\ 1 & & & & & & & \\ & & & & & & & 1 \\ & & & & & & 1 & \\ & & & & & -1 & & \\ & & & & -1 & & & \\ \end{pmatrix}$}
{$I=1$}	{$J_1=i$}	{$J_2=j$}	{$J_3=J_2^{-1}J_1^{-1}K$}
{$\begin{pmatrix} 1 & \\ & 1 \\ \end{pmatrix}$}	{$\begin{pmatrix} i & \\ & i \\ \end{pmatrix}$}	{$\begin{pmatrix} j & \\ & j \\ \end{pmatrix}$}	{$\begin{pmatrix} -k & \\ & k \\ \end{pmatrix}$}

{$\begin{pmatrix} & & & 1 & & & & \\ & & 1 & & & & & \\ & -1 & & & & & & \\ -1 & & & & & & & \\ & & & & & & & 1 \\ & & & & & & 1 & \\ & & & & & -1 & & \\ & & & & -1 & & & \\ \end{pmatrix}$}	{$\begin{pmatrix} & & 1 & & & & & \\ & & & -1 & & & & \\ -1 & & & & & & & \\ & 1 & & & & & & \\ & & & & & & 1 & \\ & & & & & & & -1 \\ & & & & -1 & & & \\ & & & & & 1 & & \\ \end{pmatrix}$}	{$\begin{pmatrix} & & 1 & & & & & \\ & -1 & & & & & & \\ & & & & 1 & & & \\ & & & -1 & & & & \\ & & & & & 1 & & \\ & & & & -1 & & & \\ & & & & & & & 1 \\ & & & & & & -1 & \\ \end{pmatrix}$}	{$\begin{pmatrix} 1 & & & & & & & \\ & 1 & & & & & & \\ & & 1 & & & & & \\ & & & 1 & & & & \\ & & & & -1 & & & \\ & & & & & -1 & & \\ & & & & & & -1 & \\ & & & & & & & -1 \\ \end{pmatrix}$}
{$J_1J_2$}	{$J_1J_3=J_2K$}	{$J_2J_3=-J_1K$}	pseudoscalar {$J_1J_2J_3=K$}
{$\begin{pmatrix} k & \\ & k \\ \end{pmatrix}$}	{$\begin{pmatrix} j & \\ & -j \\ \end{pmatrix}$}	{$\begin{pmatrix} -i & \\ & i \\ \end{pmatrix}$}	{$\begin{pmatrix} 1 & \\ & -1 \\ \end{pmatrix}$}

Alternative

We can double the dimensions initially so that we can then split them in half when we construct {$K$} and {$J_3$}. We have:

{$J_1$} as blocks of {$\begin{pmatrix} 0 & -I_2 \\ I_2 & 0 \\ \end{pmatrix}$}

{$J_2$} as {$\begin{pmatrix} 0 & 0 & I_2 & 0 \\ 0 & 0 & 0 & -I_2 \\ -I_2 & 0 & 0 & 0 \\ 0 & I_2 & 0 & 0 \\ \end{pmatrix}$}

{$K=\begin{pmatrix} -r_2 & 0 & 0 & 0 \\ 0 & -r_2 & 0 & 0 \\ 0 & 0 & -r_2 & 0 \\ 0 & 0 & 0 & -r_2 \\ \end{pmatrix}$}

{$J_3=\begin{pmatrix} 0 & 0 & 0 & r_2 \\ 0 & 0 & r_2 & 0 \\ 0 & -r_2 & 0 & 0 \\ -r_2 & 0 & 0 & 0 \\ \end{pmatrix}$} but this is {$-J_5} above.

---

Commuting with orthogonal matrices

A skew-symmetric matrix {$A$} is one such that {$A^{T}=-A$}. This is true of the orthogonal matrices {$J_i$} for which {$J_i^2=-1$}, for we have {$J_iJ_i^T=1$}, thus {$J_i^T=J_i^{-1}=-J_i$}.

Symplectic matrices {$M$} are those for which {$M^T\Omega M=\Omega$} where {$\Omega$} is a nondegenerate skew-symmetric bilinear form which we can take to be {$\Omega = J_i$}.

The subgroup of {$O(16r)$} that commutes with {$J_1$} is a subgroup of {$Sp(16r,\mathbb{R})$} and is given by {$U(8r)=O(16r)\cap Sp(16r,\mathbb{R})$}.

The subgroup of {$U(8r)$} that commutes with {$J_2$} is a subgroup of {$Sp(8r,\mathbb{C})$} and is given by {$Sp(4r)=U(8r)\cap Sp(8r,\mathbb{C})$} and this is isomorphic to the quaternionic unitary group {$U(4r,\mathbb{H})$}.

Observations

Where operators square to I, and have eigenspaces {$V_+$} and {$V_-$} of equal dimension, then these can be thought of as geodesics and also as points in the Grassmannian {$Gr_\mathbb{R}(n,2n)$}

The foursome (knowledge) is the mirror {$L$}. Knowledge evokes (just as memory does). This mirror acts by sending what is explicitly manifest (expressed symbols) to what is missing (implicit, virtual symbols - the holes of symbols) and vice versa. It is the Hackett-Kauffman sphere for the belt trick.

Ideas

• {$J_7$} yields for the sevensome a splitting of the atomic space into two parallel spaces, one of truth which keeps the eigenvector fixed, and one of falsehood which negates it. And then {$J_8$} identifies these two parallel spaces, negating them.
• {$Cl_{0,6}\cong R(8)$} models 6 perspectives, the most we can introspect, and to go beyond that we would need larger matrices and we can't.

Linear complex structures

• {$J_1$} is taken to be the block diagonal matrix with {$2\times 2$} blocks {$b_1$}. It defines what is unitary.
• {$J_2$} is taken to be the block diagonal matrix with {$4\times 4$} blocks {$b_2$}. It further defines what is symplectic.
• {$J_3$}
• {$J_4$}
• {$J_5$}
• {$J_6$}
• {$J_7$}
• {$J_8$}