Introduction E9F5FC

Questions FFFFC0

• View
• Edit
• History
• Print

A math result is an Alexandrian pattern which brings together:

• the forces in tension: the axioms or principles that it involves
• the instances, particular and general: the objects (of whatever degree of abstraction) that it holds for
• the logic that holds it together, the rule of thumb: an argumentation (a proof or demonstration)
• the way of figuring things out that brought it together

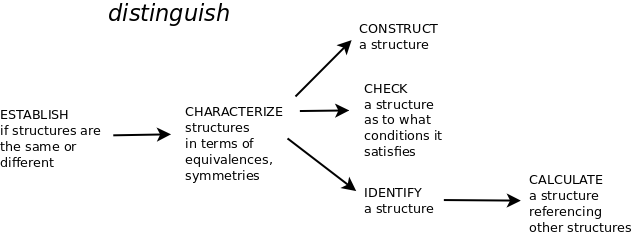
Counting: characterizing a set.

Note: the action becomes an object in its own right. For example, an object is characterized by whether it is an instance of itself or is not. The multiset of instances is then characterized by distinguishing a set of distinct instances. A set is characterized by counting it. But the count is not a set but a list. In this way, algebra unfolds to yield counting.

Study polymorphism of principles of basic math

Math should derive from logic, from perfect duality, and variation from it. The relation between logic and metalogic is given by our interpretation of a variable with reference to one of four levels. This establishes an analogy between logic, set theory, category theory and...? See logic.

List basic principles of math:

• Every answer is an amount and a unit. These are the grounds for recognizing differences (amounts) and similarities (units). "Comparing apples and oranges."
• Combine like units.
• List unlike units.
• Extend the domain.
• Every right triangle is half a rectangle.
• Every triangle is two right triangles.