Introduction E9F5FC

Questions FFFFC0

• View
• Edit
• History
• Print

Lie Group Embeddings

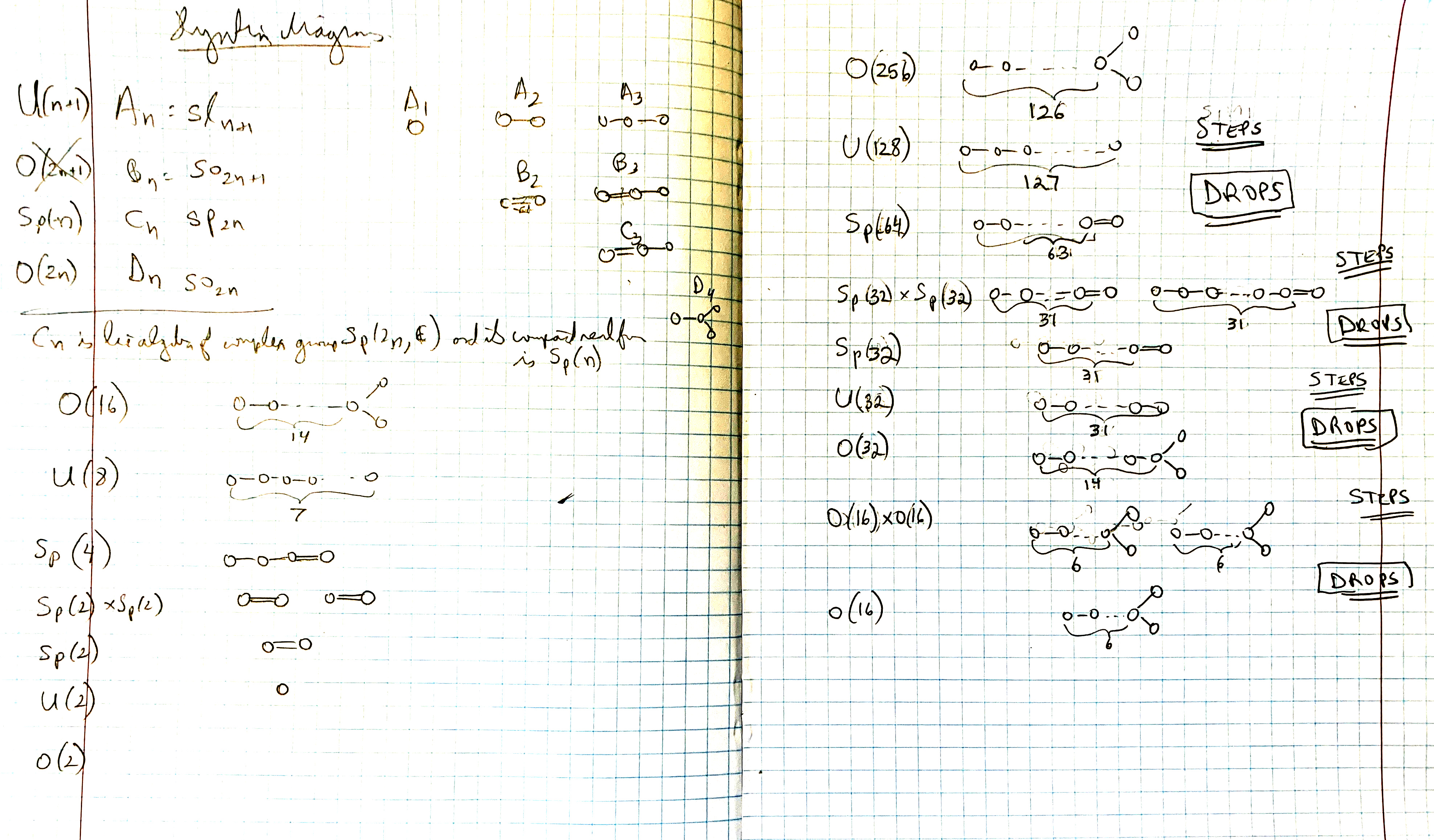
{$O(16r)\supset U(8r)\supset Sp(4r)\supset Sp(2r)\times Sp(2r) \supset Sp(2r) \supset U(2r) \supset O(2r) \supset O(r)\times O(r) \supset O(r)$}

Note that we can start anywhere. We simply forget the previous complex structures. Thus the division of everything incorporates a new perspective, yielding a new division of everything, by way of the operation +1, or similarly, +2 or +3.

---

{$O(16)\Rightarrow U(8)$}

O2

Write the linear complex structure {$J_1$} as:

{$J_1=\begin{pmatrix} 0 & -1 & & & \\ 1 & 0 & & & \\ & & 0 & -1 & \\ & & 1 & 0 & \\ & & & & \ddots \end{pmatrix}$}

What orthogonal matrices {$O$} commute with {$J_1$} so that {$OJ_1=J_1O\;$}?

Multiplying out we see that we get {$2\times 2$} blocks which express complex numbers.

{$\begin{pmatrix} a & -b \\ b & a \end{pmatrix}$}

Rotations commute and rotoreflections do not.

When we have four linear complex structures {$J_\alpha, J_\beta, J_\gamma, J_\delta$}, any two of them yield a shift in perspective (a quaternionic structure), and any three of them ground three-cycles on a splitting of the vector space. Putting that together we may have possibly

---

{$U(8)\Rightarrow Sp(4)$}

What unitary matrices {$U$} commute with {$J_1$} so that {$UJ_1=J_1U\;$}?

{$\begin{pmatrix} x & y \\ -\bar{y} & \bar{x} \end{pmatrix}$}

---

{$Sp(4)\Rightarrow Sp(2)\times Sp(2)$}

{$\begin{pmatrix} Sp(2) & 0 \\ 0 & Sp(2) \end{pmatrix}$}

---

{$Sp(2)\times Sp(2)\Rightarrow Sp(2)$}

{$\begin{pmatrix} Sp(2) & 0 \\ 0 & \textrm{same} \end{pmatrix}$} with blocks {$\begin{pmatrix} x & y \\ -\bar{y} & \bar{x} \end{pmatrix}$}

---

{$Sp(2)\Rightarrow U(2)$}

{$\begin{pmatrix} U(2) & 0 \\ 0 & \textrm{same} \end{pmatrix}$} with blocks {$\begin{pmatrix} x & 0 \\ 0 & \bar{x} \end{pmatrix}$}

---

{$U(2)\Rightarrow O(2)$}

{$\begin{pmatrix} O(2) & 0 \\ 0 & \textrm{same} \end{pmatrix}$} with blocks {$\begin{pmatrix} a & 0 & 0 & 0 \\ 0 & a & 0 & 0 \\ 0 & 0 & a & 0 \\ 0 & 0 & 0 & a \end{pmatrix}$}

---

{$O(2)\Rightarrow O(1)\times O(1)$}

{$\begin{pmatrix} O(1) & 0 & 0 \\ 0 & O(1) & 0 \\ 0 & 0 & \textrm{same} \end{pmatrix}$} with blocks {$\begin{pmatrix} a & 0 & 0 & 0 \\ 0 & a & 0 & 0 \\ 0 & 0 & a & 0 \\ 0 & 0 & 0 & a \end{pmatrix}$}

Note that {$O(1)=\{(1),(-1)\}$} so {$a=1$} or {$a=-1$}. But also, we could have started with {$O(16r)$} and we could have here {$O(r)$}.

---

{$O(1)\times O(1)\Rightarrow O(1)$}

{$\begin{pmatrix} O(1) & 0 & 0 \\ 0 & \textrm{same}\; O(1) & 0 \\ 0 & 0 & \textrm{same}\;O(1)\times O(1) \end{pmatrix}$} with blocks {$\begin{pmatrix} a & 0 & 0 & 0 \\ 0 & a & 0 & 0 \\ 0 & 0 & a & 0 \\ 0 & 0 & 0 & a \end{pmatrix}$}

{$U(2r) \supset U(r)\times U(r) \supset U(r)$}

We define transformations {$K_1=iJ_1$} and {$K_2=iJ_2$}. {$K_k^2=I$}, {$(K_k+1)(K_k-1)=0$}, {$(K_k+1)(K_k-1)v=0$}, so {$K_kv=v$} or {$K_kv=-v$}. {$K_k$} thus splits {$\mathbb{C}^{2r}$} into two eigenspaces.

Note that {$J_kv=-iv$} or {$J_kv=iv$}. In other words, the eigenvalues of {$J_k$} are {$\pm i$}.

{$U(2)\Rightarrow U(1)\times U(1)$}

Consider the effect of {$J_1$} on {$J_1v$}. We have {$J_1^2=-1$} thus {$J_1(J_1v)=-v$}.

Suppose {$v$} is such that {$J_1v=-iv$}. Then {$J_1(J_1v)=-iJ_1v$} and thus {$J_1v$} is in the same eigenspace as {$v$}.

Likewise, suppose {$v$} is such that {$J_1v=iv$}. Then {$J_1(J_1v)=iJ_1v$} and thus {$J_1v$} is in the same eigenspace as {$v$}.

Thus {$J_1$} does not take us from one eigenspace to another. This is because {$i$} is a scalar.

Suppose a unitary matrix {$U$} commutes with {$J_1$}, which is to say, {$UJ_1=J_1U$}.

Suppose {$v$} is such that {$J_1v=iv$}. What can we say about {$Uv$}? {$J_1Uv=UJ_1v=Uiv=iUv$}.

Similarly, suppose {$v$} is such that {$J_1v=-iv$}. Then {$J_1Uv=UJ_1v=U(-iv)=-iUv$}.

Thus {$U$} respects the eigenspaces. If the eigenspaces are the same size, then {$U\in U(1)\times U(1)$}.

Can we say, conversely, that {$U(1)\times U(1)$} consists of all of the unitary matrices that commute with {$J_1$}?

{$U(1)\times U(1)\Rightarrow U(1)$}

Shintaro Fushida-Hardy. Notes for MATH 282B Homotopy Theory. Explains fibrations and basic examples of fibre bundles involving Lie groups and also projective spaces. Also talks about loop spaces.

{$$\mathbb{S}^0\hookrightarrow \mathbb{S}^n\hookrightarrow \mathbb{R}\mathbb{P}^n$$} {$$\mathbb{S}^1\hookrightarrow \mathbb{S}^{2n+1}\hookrightarrow \mathbb{C}\mathbb{P}^n$$} {$$\mathbb{S}^3\hookrightarrow \mathbb{S}^{4n+3}\hookrightarrow \mathbb{H}\mathbb{P}^n$$}

{$$\mathbb{C}\mathbb{P}^1\cong\mathbb{S}^2=\mathbb{C}\cup\{\infty\}$$}

{$$\mathbb{S}^1\hookrightarrow \mathbb{S}^{3}\hookrightarrow \mathbb{S}^{2}$$}

{$$0=\pi_3(\mathbb{S}^1)\to\pi_3(\mathbb{S}^3)\to\pi_3(\mathbb{S}^2)\to\pi_2(\mathbb{S}^1)=0$$}

thus {$\pi_3(\mathbb{S}^2)\cong\pi_3(\mathbb{S}^3)=\mathbb{Z}$}

{$$\mathbb{S}^3\hookrightarrow \mathbb{S}^{7}\hookrightarrow \mathbb{S}^{4}$$}

{$$O(n-1)\hookrightarrow O(n)\hookrightarrow \mathbb{S}^{n-1}$$}

{$$U(n-1)\hookrightarrow U(n)\hookrightarrow \mathbb{S}^{2n+1}$$}

{$$Sp(n-1)\hookrightarrow Sp(n)\hookrightarrow \mathbb{S}^{4n+1}$$}

thus {$\pi_k(O(n-1))\cong\pi_k(O(n))$} for {$k<n-2$}.

Grassmannian {$G(n,k)=G_n(\mathbb{R}^k)$} is the {$n$}-planes in {$\mathbb{R}^k$}

{$V(n,k)=V_n(\mathbb{R}^k)$} are the orthonormal sets of size {$n$} in {$\mathbb{R}^k$}

Suppose {$n < m < k$}.

{$$O(n)\hookrightarrow V(n,k)\hookrightarrow G(n,k)$$}

{$$OV(m − n,k − n)\to V (m, k) \to V (n, k)$$}

{$$V(m − 1, k − 1) \to V(m, k) \to S^{k−1}$$} when {$n=1$}

Thus {$V(m, k)$} is {$(k − m − 1)$}-connected. Consequently, {$V(m, \infty)$} is weakly contractible.

From our sequence {$O(n)\hookrightarrow V(n,k)\hookrightarrow G(n,k)$} we have that {$\pi_n(G(m,\infty))\cong\pi_{n-1}(O(m))$}, yielding {$G(m,\infty)\cong BO(m)$}.

Note that for {$O(2n)\supset O(n)\times O(n)$} we have not just that {$O(n)\times O(n)$} are the matrices that commute with {$J_7$}, but also that there exist mutually anticommuting {$J_1,J_2,J_3,J_4,J_5,J_6$} which anticommute with {$J_7$} and commute with {$O(2n)$}. This means that {$O(2n)\supset U(n)\nsupseteq O(n)\times O(n)$} for the other linear complex structures constrain {$J_7$} so that it does not commute with all of {$U(n)$} but only part of it.

Analyzing the dimensions

• {$O(n)$} has {$n(n-1)/2$} real dimensions
• {$U(n)$} has {$n^2$} real dimensions
• {$Sp(n)$} has {$n(2n+1)$} real dimensions
• {$O(16)$} has {$120$} real dimensions; {$U(8)$} has {$64$}; {$Sp(4)$} has {$36$}; {$Sp(2)\times Sp(2)$} has {$20$}; {$Sp(2)$} has {$10$}; {$U(2)$} has {$4$}; {$O(2)$} has {$3$};{$O(1)\times O(1)$} has {$0$}; {$O(1)$} has {$0$}.
• {$O(4)\times O(4)$} has {$12$} real dimensions.

Karoubi: The classical groups are built from spheres as fibrations: {$O(n)\rightarrow O(n+1)\rightarrow S^{n}$} and {$U(n)\rightarrow U(n+1)\rightarrow S^{2n+1}$}. From these fibrations it immediately follows that the homotopy groups of {$O(n)$} and {$U(n)$} stabilize.

{$\pi_i(O(n))\cong\pi_i(O(n+1))$} for {$n>i/2$} and {$\pi_i(U(n))\cong\pi_i(U(n+1))$} for {$n>i+1$}

Using polar decomposition of matrices, in the Bott periodicity theorem, we can replace {$O(n)$} and {$U(n)$} and {$Sp(n)$} with {$GL(\mathbb{R})$} and {$GL(\mathbb{C})$} and {$GL(\mathbb{H})$}, respectively.