John Harland leads the Math 4 Wisdom study group for
Andrius Kulikauskas presents:
John Harland's Research Program
Goal
John Harland seeks to contribute to a more solid or plausible ontological foundation for physics.
Questions for John
- What are the problems in the foundations of physics that trouble you?
- Why is a distinction between actuality and possibility not satisfactory?
- How would you distinguish between classical physics and quantum physics and how would you relate them?
- Collapse of the wave function
- Agency hierarchy
- Retroactivity and superluminality
- Factor of 2
- Culling and editing histories
- Evolution of... states? the physical constants? the laws of physics?
- Related theories? Penrose
Research Question
Does an apparent time factor of {$\frac{1}{2}$} arise in comparing classical and quantum unitary representations of relativistic dynamical systems?
Key Concepts
Evolution
Learnability
Agency hierarchy
Culling
Superluminality
Editing histories
Backwards and forwards
Unitary
Relativity
Local and nonlocal
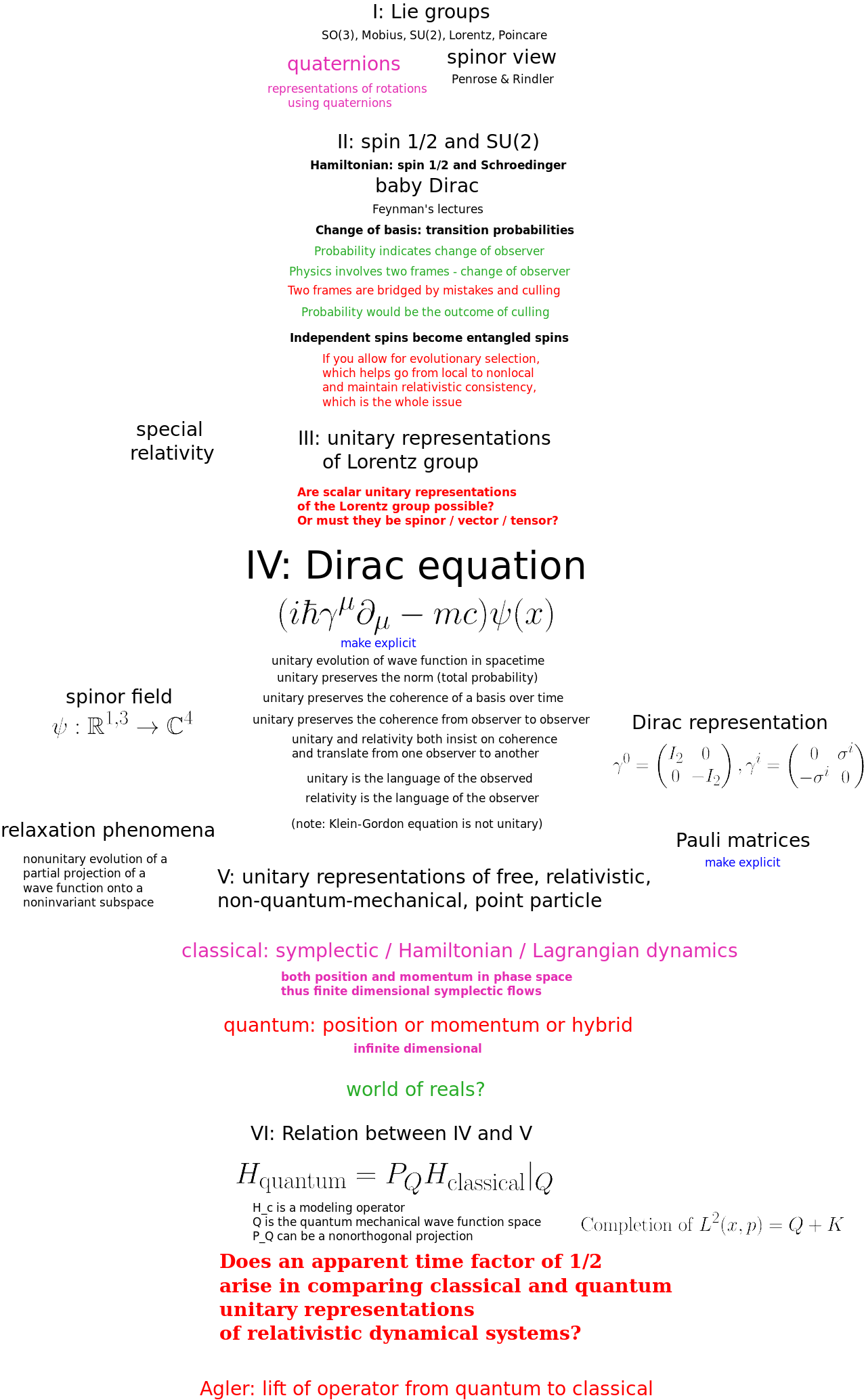
Progression of Topics
I. Lie Groups
II. Spin {$\frac{1}{2}$} and {$SU(2)$}
III. Unitary representations of Lorentz group
IV. Dirac equation
V. Unitary representations of free, relativistic, quantum-mechanical, point particle
VI. Relation between IV and V
VII. Time factor
Does an apparent time factor of {$\frac{1}{2}$} arise in comparing classical and quantum unitary representations of relativistic dynamical systems?
Additional notes by Andrius Kulikauskas about John Harland's thinking