• View
• Edit
• History
• Print

Research program, 20220128 Research Program Introduction, Research program overview, Research program God

---

---

Introduction to Math 4 Wisdom

This is most of the transcript for my video Introduction to Math 4 Wisdom.

I am in the process of adding all of the text and also screenshots.

---

---

---

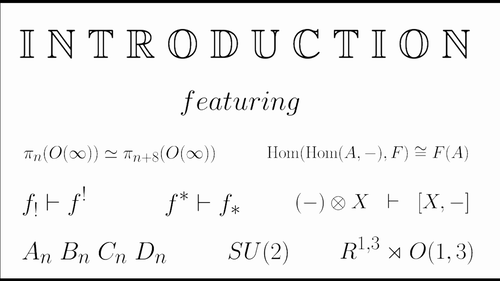
Math 4 Wisdom relates the language of wondrous wisdom and the language of advanced mathematics.

This introductory episode of Math 4 Wisdom is brought to you by the wondrous wisdom of One, All, Many.

Sponsor: When you're looking for One, you might just find All, and you're making the most of Many.

---

---

Mu: That's what I've been saying with advanced mathematics. I am the Minimization operator Mu. I am looking for One. I may go through All. I have gone through Many.

Announcer: Minimization operator Mu, there is an empty spot for you in this episode in approximately seven minutes.

Mu: Hang on til I find it!

---

---

Question: Why math?

Answer: Math for wisdom.

Question: Why wisdom?

Answer: Wisdom knows why.

Question: Why why?

Answer: Why "why why?"?

Question: Why why why why why why why why why ... ?

Announcer: Never mind Why, here's Math 4 Wisdom!

In this video, I introduce Math for Wisdom. I overview my research program. You may help me and learn along with me. I welcome your support through Patreon.

My name is Andrius Kulikauskas. I am speaking from my home in Lithuania. Please visit my website, Math4Wisdom.com, where you can read the text for this video, observe my research activities, collaborate with me and others, and contact me.

I am learning, studying, exploring and discovering advanced mathematics at a graduate student level which I personally find relevant in my lifelong quest to know everything and apply that knowledge usefully.

Specifically, I want to learn enough algebraic topology to intuitively understand Bott periodicity inside out. In category theory, I want to master the Yoneda lemma and I want to classify adjoint strings. In algebraic geometry, I want to understand derived functors and sheaf theory so that I could intuit Grothendieck's six functor formalism. As regards Lie theory, why are there four infinite families of Lie groups and Lie algebras? How might they lay the foundations for four geometries - affine, projective, conformal and symplectic? I wish to master the special unitary groups SU(2) and SU(3), including their gauge theories. And I seek a deep understanding of the Poincare group, the isometries of Minkowski space-time.

All of these topics have in common my wish to explore and express mathematically what is a perspective? and how do perspectives fit together?

A perspective is the difference between relative truth and absolute truth. How can I get free of my own perspective? I can allow for other perspectives, I can appreciate the frameworks by which perspectives fit together, and I can learn what it means to have no perspective at all. In such ways, I can think holistically, which is to say, think and know in terms of everything. Wisdom is truth about everything.

I will give an example of how three different perspectives fit together. In this example, I will define three concepts: one, all and many. I am not aware of logicians ever defining these concepts. Here is my definition.

If you're looking for One, you might just find All and you're making the most of Many.

Concentrate. Help me concentrate. Wondrous Andrius help me concentrate!'

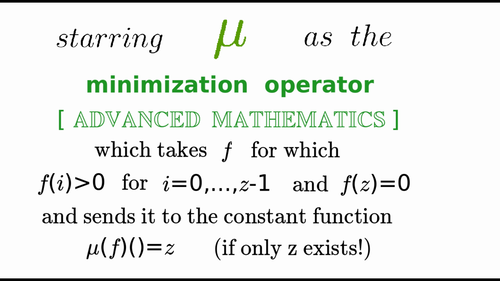
One, All, Many are three mental circumstances which are employed in computability theory, specifically, in defining the minimization operator μ, which is a schema for constructing partial recursive functions, also known as general recursive functions.

These are set functions whose domain and codomain are the natural numbers, by which I mean the nonnegative integers. Indeed, their inputs and outputs can be understood to encode finite lists of natural numbers.

Our story begins with primitive recursive functions, which are generated by five schemas: the successor function, constant functions, projection functions, the composition operator and the primitive recursion operator.

Primitive recursive functions are total functions, which means that they are well defined everywhere. But this well definedness actually limits their power to compute.

A sixth schema, the minimization operator μ, is needed to achieve the full power of computing as declared by the Church-Turing thesis, by which Turing machines, the lambda calculus and general recursive functions (and other such) all exhibit this ultimate power.

PRF: You are willing to suppose that this opportunistic operator mu goes through each of the many, out to infinity, never halts, and then will come back to us to certify that he found nowhere to park his seeds. That's the convoluted way you're going to dream up the indefinite? Why don't you just establish an indefinite constant function without this dogma of coming back from infinity?

C: An indefinite constant function would be out of the scope of our successor function. Operator mu is fundamentally inverting everything we've ever done. The indefinite testifies to that.

PRF: Mu doesn't have any runners to maintain links and keep track of what he's doing, does he? Maybe you feel that we, primitive recursive functions, are too rigid, too controlling?

The minimalization operator μ does this by bringing together three mental circumstances which unfold in a search for constancy, namely, One, All, Many. Indeed, we can think of minimization as a search for constancy, a search for zero, a search for whatever zero could mean. We will see that zero can mean one or all or many, wherever the constancy should be.

Let f(n) be a primitive recursive function, or more generally, a general recursive function. Then the minimization operator μ defines a function μ(f) whose output may be defined or undefined but depends only on f and is independent of n, the input for f. μ(f) returns, if possible, the least value n such that f(n)=0 and f(m) returns a nonzero number for any m<n. If there is no such n then μ(f) does not return anything, does not output anything, but is undefined and we can say that it never halts but runs forever.

The way to think about the minimization operator μ is that it is an algorithm which constructs μ(f) from f by calculating f(0) and then f(1) and then f(2) and then f(3) and so on as if they were subroutines. Running any of these subroutines f(i) yields various possible outcomes.

• First, it may be that f(i) outputs 0 and assuming that we have been proceeding methodically, we have found the least such i and so we set μ(f)=i and we are done.

Mu: My parking spot is the very first available, which makes me minization operator mu. Now I print out mu of f, a constant function that gives the number where I parked, so I never forget the one spot I took. One and only one. I am perfect parker.

• Second, if f(i) outputs a nonzero number, then we continue by running f(i+1).

Mu: I am so methodical. I check to see, is the spot is taken? And if it is, I move on to the next spot. I have my method of many spots! I am perfect parker.

Those are the two possibilities if f is a total function, defined for all natural numbers.

• But if f(i) is undefined, then μ(f) is likewise undefined and we are done.

Mu: I got stuck at this spot to see if it was free but it was undefined. I thought this car was leaving but they couldn't figure out what they were doing. They took forever. Forever! Total gridlock! So I print out mu of f, a constant function that is undefined, without a number, to certify no parking. I am perfect parker.

• But even if f is a total function which is always defined, then if there is no i for which f(i)=0, then there is no least such i and μ will run forever, will never halt and μ(f) will be undefined.

Mu: I have gone through all spots, out to infinity, and the parking lot is totally full. So I print out mu of f, a constant function that is undefined, without a number, to certify no parking. I am perfect parker.

In this way the minimization operator μ takes a function f that may be defined for all n, and constructs a function μ(f) that may be undefined for all n. Basically, we are constructively inverting the function f to identify the least value i for which f(i)=0. But there may be no such i, in which case μ(f) is undefined.

The point of this construction for us is that it showcases the concepts One, All and Many.

Imagine that the number 0 represents constancy and that we are searching for constancy. Either we find a least i which give us 0, in which case we have found one example of constancy. Or there is no such i, in which case it is all constantly unconstant, as the constancy is in All. But furthermore we have been leveraging a third form of constancy, inasmuch as input i does yield an output f(i). In the series of subroutines, we constantly assume that if a subroutine halted and returned output, then we must have run it and provided it input. This is the regularity of the many by which we can say those subroutines are multiply constant.

This suggests that in addition to the existential quantifier and the universal quantifier we could have a multiplicial quantifier, an upside down M, to make explicit that a variable n, as an input, yields well behaved outputs, is being used constructively, has a type, can be compiled, or something anything like that.

Let us consider now how the number zero can mean One, All or Many. Zero is the output we are looking for, and it can refer to the input i as the One that produced it.

Plants: Did you hear that, superhero? You're a definite loser!

Mu: Sometimes I win.

Plants: What do you win, super zero?

Mu: I win zero.

Plants: Zero is nothing. Zero is for losers.

Mu: Zero is a placeholder for nothing. That's the difference between one Swiss franc and one quadrillion Swiss francs.

Wondrous Andrius: Zero is also the unconditional nonexistence of such an i, and in that sense, zero refers to all.

Plants: Wow, that's when you're a total loser!

Plants: Yeah, total loser!

Plants: You can't answer that! Can you?

Mu: The indefinite is more powerful than the definite. The indefinite doesn't have to give an answer. It's what makes the full fledged Turing machine the most powerful in the Chomsky hierarchy because it has the power to erase, delete, make a variable disappear, make a construction irreversible, untraceable. Irreversibility gives the second law of thermodynamics its power for dooming the world and for creating life. The indefinite has Godly power. But you are total, you are all definite. None of you are partial, none of you are indefinite. You always have an answer, you can't not have an answer. So you don't understand.

Wondrous Andrius: And zero is the conditional regularity of the Many by which an input gives rise to an output, and there is no obstacle to hinder that.

Plants: You're not just a loser, you're a subloser! You're a gambler!

Mu: I am a player. I keep playing.

Plants: You keep waiting to win. And if you win, you win zero. And mostly you're just losing, over and over, you don't find your zero. And you may never win!

Mu: The indefinite goes beyond itself into the definite and they sort out the truth, they match conscious question and unconscious answer, and consciousness manages them, piece by piece, subroutine by subroutine. Consciousness forms the many.

Announcer: By gambling you can lose not just your many, but your wife's many, your children's many, your friends' many, your many jobs, your many homes, your many cars, your many pets, your many plants and your many routines that make up your hu-many-ty.

Mu: Many makes life real. Many is the basis for counting. When we count forwards we also count backwards by duality. Understanding that forward-backward symmetry distinguishes classical Lie algebras.

Mu (with goggles): Now I am going deep and murky, turkey. The root system of the Lie algebra of the unitary group is a straightforward chain. So winning is losing and losing is winning. We can fold the chain, replace the complex norm with a quaternion norm, for the symplectic group. We can cut the chain and fuse the ends so that one equals negative one for the even dimensional orthogonal groups with a real norm. Or we can connect both ends to an external zero for the odd dimensional orthogonal groups. That's how we get zero for counting. Counting backwards to zero is life which ends in death and counting forwards towards infinity is eternal life. Let us count forwards with zero, not look backwards to zero. But you don't understand.

Plants: Don't tell us about root systems! Get a real job! Grow something!

Wondrous Andrius: We thus make sense of zero in these three mental circumstances which give meaning to constancy.

Plants: You're a constant all-around loser!

Mu: Yes I am a definite loser, indefinite loser, subloser. That's how I find a crack to plant a seed. I'm looking for a crack, baby! I have a special understanding of zero.

Plants: Superzero, while you've been waiting to find your zero, biologically speaking, we've been dying in anticipation!

Announcer: Real plants died in the making of this video.

Plants: That's not funny! Get us some sparkling water! Puhleaze!

I have simplified my explanation of the minimization operator μ by neglecting or delaying to say that, in general, f may have additional inputs {$x_1,...,x_k$}, in which case μ(f) will be a function of these inputs, and so, for different values of these inputs it may be undefined or not and it may produce different outputs. I stepped around this by supposing that the list of inputs could be coded as a single natural number.

In this introductory video, I have elaborated the foundations for the concepts of One, All, Many so that you could be interested to investigate the relationships between mathematics and metaphysics or cognition, and more broadly, wisdom.

In my videos, I will share what I know, but you will need to study independently, and so I encourage you to do exercises. I write out technical terms so that you could look them up. When you are reading Wikipedia articles, watching related videos, studying books and papers, and thinking, talking and writing about these issues, then you are doing exercises in Math 4 Wisdom. At Patreon you can find ways that I or others can give you feedback and you may even win prizes when you do exercises. My primary goal with Math 4 Wisdom is to encourage a community of investigators of math for wisdom.

Would you like to learn more about the minimization operator μ ? I refer you to the Wikipedia article on "general recursive functions" and also the video series on partial recursive functions by Hackers at Cambridge.

[Origin stories]

Back in 1986, as a senior at the University of Chicago, I took two graduate level courses in recursive function theory taught by Robert Soare, who published his lecture notes as this book I am holding.

In 1993, while pondering how to define twelve mental circumstances, including One, All, Many, I recalled the spirit of the arguments we used, and expressed it metaphysically. In 2022, in preparing this video, I was surprised and delighted to realize that this metaphysics is captured in the very first pages by the minimization operator μ, which Soare called unbounded search.

Mu: Unbounded search. I like that!

If you are like me, then you may wonder how profound are the six schema? Do they have any cognitive, conceptual or metaphysical significance? Mathematically, there are lots of ways of cooking up schemas that will yield the general recursive functions. Nevertheless, if we express and organize these schema in a most elegant way, then we may reveal underlying structural symmetries that may be unavoidable.

In particular, we could try to relate these six schema to the levels of the Chomsky hierarchy - finite automata, pushdown automata, linear bounded automata and Turing machines. If you would like to investigate such problems together, then let me know. At my Patreon page you can learn how I could advise you, a bit like an advisor for a PhD student.

Pedagogically, I love to show how ideas branch out and how they relate, but I believe in these videos it is important to keep returning to the main ideas so that you can approach them as many ways as possible, including emotionally.

The minimization operator μ does not define One, All, Many in terms of simpler concepts but rather acts as a mind game by which we demonstrate our familiarity with these concepts.

Let us now make this fun and memorable and practical with a real life problem.

You are a princess and you are looking for a prince, the love of your life. You disguise yourself as a traveling salesperson, which is how everybody has known you since birth. You proceed methodically, house by house, starting with the first house on a street that extends on to infinity. At each house you ring the doorbell and you wait for the door to open. If your prince opens the door, then you have found the One. But maybe a frog opens the door, so you go on to the next house, and so on, and so on, and that is All. And then there is the assumption that you realize you have been making, which is that the doorbell you ring is actually related to the particular door that opens, because otherwise your plan doesn't make any sense. Your plan assumes the Many.

So that shows that you know what it means to be One or All or Many.

But it also shows that you may be ringing doorbells forever, which means that you are never at home. And maybe your prince is looking for you but you are not opening your door.

Who should be looking for who? That is the question!

Then you realize that when you get to your house you may find the prince there. But then you realize that you may get to his house first and he will never answer because he is never at home. And you will both wait at each other's doors forever. But actually, if you both are out ringing doorbells on the same street, patiently and methodically, as befits true lovers, then you will find each other at the same door, the first door which never opens, as you wait there forever, in endless anticipation, happily ever after. The End.

Let us search for constancy. Either we find one example of constancy or we don't. This defines one. If we don't find an example, then all is constantly unconstant. This defines all. And finally, our search assumes that the thing we choose to examine and the thing we make conclusions about are indeed the same thing. This regularity must hold for the many and so defines it.

If you are familiar with computability theory, as in the study of Turing machines with oracles, then you will recognize this argument. It is part of mathematical thinking, what goes on in the mind yet often doesn't get written down on paper, perhaps because we don't know how to formalize it, axiomatize it or otherwise acknowledge it. A general theme in Math 4 Wisdom is to slow down our thinking, to appreciate the steps that we take in our mind, to delineate the possiblities and to recognize how limited they are, as regards our imagination.

If you and I go through such mental motions together, then we share a journey through a model of an abstract mind, which is a basis for shared understanding, and pragmatically, for absolute truth. Since 1982, when I was seventeen, I have been observing, collecting and assembling such cognitive frameworks. I have developed what you might call my own private language. I believe that it is a language of wisdom. But why should anybody care? And who wants to think so abstractly?

With Math 4 Wisdom, I am relating my language of wisdom with the language of mathematics. On the one hand, this will help me express wisdom accurately and draw insights from mathematics. On the other hand, I aspire to show that wisdom lets us identify the most profound ideas and structures in mathematics, and furthermore, overview all of mathematics, understand how its disparate branches, concepts, theorems, questions, structures arise and unfold. I seek wisdom that teaches us how to formulate any question, solve any problem, pursue any conjecture, understand any idea.

In the upcoming videos, I will present the main parts of my research program. First, I will introduce the ABC's of thinking-in-parallel, which is to say, the three most important mental frameworks, which I call the twosome, threesome and foursome. Next, I will show how they fit within a system of eight mental frameworks, which relates to my interest in Bott periodicity. Then I will survey the ways of figuring things out in mathematics. After that, I will show how the 24 ways provide a map of mathematical thinking. In particular, I will explain why I think there are four geometries and why I think the Poincare group relates them. Finally, I will overview the many structures that I am familiar with, and sketch a theory of how I think they all fit together, and mention a coincidental relationship to the Standard Model in particle physics, which suggests that the gauge theory for gravity has the form of 0 by 0 matrices, whatever that could mean.

That last video in this introductory series will lay out my current understanding as to the meaning of life and the role of God, I, You and Others. I think I should tell you the key points now so that you would have a sense of my outlook, what I think is wisdom and the ways in which God is relevant. It will help you understand who I am and why I am creating Math 4 Wisdom.

[Feedback]

Mu: Sounds like feedback.

[Pick up mail from "email" box]

[Antonio Jesus 47:30 I would not hit the table.]

Andrius under table: Why did I hit the table? I want to get the attention of viewers like you. Why do I want your attention? I want you to be conscious of wondrous wisdom and what it means for our lives and how we should all work together with a language, a science, a community, a civilization, a universe of wondrous wisdom for all. We should live eternal life here and now!

Mu: Your viewers are helping you!

[Viewers like you. Rimas Morkūnas]

Mu: They appreciate what you are trying to do. They are giving you attention. They are learning with you.

Andrius: Some of them are liking and subscribing and telling their friends and supporting me through Patreon and showing faith in all of us in Math 4 Wisdom Land.

Mu: That's what you want! You don't want to live under the table. You want kitchen talk.

Andrius: What is kitchen talk?

Mu: Math 4 Wisdom Land Kitchen talk is when you get from under your table and you talk to friendly people in Math 4 Wisdom Land about what you really care about, which is

Together: Math 4 Wisdom

[Math 4 Wisdom Land Kitchen Talk with John and Thomas]

[From the roof]

In today's episode I provided a basic example of how three mental circumstances - one, all, many - appear in advanced mathematics in the minimization operator mu. Why am I obsessing over this example of the link between mathematical thinking and wondrous wisdom or mental frameworks or metaphysical structures or whatever you'd like to call it?

[Foot on the ladder]

Think of this basic example as a tiny data point from which we'll zoom upward to get the big picture of wondrous wisdom. Rhetorically, it's like the base case of mathematical induction in that once you accept that advanced mathematics can get your foot on the ladder of wondrous wisdom, then you may further believe that advanced mathematics can help you climb up it as far as it goes.

[Show the chest of tools]

Have you never heard of mathematical induction? It is one of the keys to mathematics, or more precisely, one of 24 ways of figuring things out in mathematics, which our mind makes use of. I systematized such ways by studying the patterns in the books by George Polya, Paul Zeitz and others. Indeed, I have collected more than one thousand examples of ways of figuring things out not just in mathematics but in many other fields of life. This is a perfect activity for us all to collaborate so that we can survey and compare the epistemologies of scientific disciplines and creative personalities and thereby appreciate how and why the frameworks of wondrous wisdom arise inevitably. Your support through Patreon make this all the more possible for the disciplines that you personally care about. I look forward to making videos not just about the keys to mathematics but also other areas for which I have sketched them out including biology, neuroscience, physics, chess, the business innovation games known as Gamestorming, and also the ways of figuring things out used by Jesus, used by the Gaon of Vilna and that I myself used in exploring wondrous wisdom.

What was I saying?

Mu: Where will the ladder take us?

Please, if I may, for the next five minutes,

[Start to sing]

break out in song, technically and speculatively,

• One, all, many are one of four conceptions of the threesome, the learning cycle of taking a stand, following through, and reflecting.
• The threesome is one of eight divisions of everything, which suggests Bott periodicity in advanced mathematics.
• The divisions are one of 24 structures that channel the flow of experiences in the worldview of me, me, me, me the first person.
• I am one of four persons with worldviews by which we imagine all that we imagine, a zeroth person God, a first person I, a second person You, a third person Other.
• We can allow for more than we imagine, as the Indefinite goes beyond itself into the Definite and then inverts that for the Imagineable and finally emerges anew as the Unimagineable. This is the most basic understanding how God goes beyond God into us in these four stages. Can we understand this more compactly?
• Yes! Yes! Yes! in three stages with self-understanding
• Yes! Yes! in two stages with shared understanding
• Yes! in one stage with good understanding!
• What is this good understanding? Good understanding is the pinnacle of wondrous wisdom.

[Flying and singing]

The wisdom of the lost child. She has self-understanding, that she is lost. She has shared understanding, that her parents care that she not be lost. She has good understanding of the asymmetry of childhood and parenthood, that she is the child and they are the parents, so she should not look for them but they should look for her, so she should go wherever they will find her.

[New environment... paths in the forest... from three perspectives]

Good understanding is amazing in that child and parent, or analogously, human and God, are able to coordinate their activity without any channel of communication. The wisdom of the lost child is the complete unfolding of childish understanding as the child's view of the parents' view of the child's view of the parents' view of the child's view. In this way, the child is able to coincide with the parents's view and thereby give up their own childish perspective, which is the point of consciousness, to let go of ourselves.

In a future episode I will survey for you in more detail what I am able to say of this wondrous wisdom, which is my life's work, but truly should be collaborative. I am a lost child and perhaps you are, too. I have a sense of the big picture of the life we live and perhaps you do likewise. Math 4 Wisdom is my endeavor to work together as a scientific community to show where the holistic frameworks of wondrous wisdom, relevant for modeling and understanding the big picture, can be found in the practice of advanced mathenatics, which reflects the limits of mathematical imagination.

I will make many videos about the various cognitive frameworks and where they arise in advanced mathematics. Sometimes I will craft a video as a Math 4 Wisdom Show like this one. At other times it will be unscripted as with Kitchen Talk. I will also post dozens of academic talks that I have given about these frameworks. And I will regularly update you on the insights I am gaining in my mathematical investigations to observe, develop and apply wondrous wisdom, and such updates will get quite technical.

[In bed...]

My quest to know everything makes it helpful to imagine God's point of view. I will be creating a series of videos to share the various ways I imagine God and will try to model God with advanced mathematics. For example, I think of God as a state of contradiction in which all things are true, and I think of God's activity as his or her going beyond himself to give rise to a state of noncontradiction. I am curious if one model for God is the mythical field with one element, which is to say, a field where zero equals one equals infinity. When I read in Wikipedia that the action of the anharmonic group on zero, one and infinity gives an isomorphism with {$S_3$}, as the quotient of {$S_4$} over the Klein group, then I wonder, what does that say about God?

Speculations about God are for me the top down approach which develops a theory to make sense of the facts accumulated by the bottom up approach of documenting cognitive frameworks such as one, all, many. Connecting theory and facts requires a personal resolve to apply that knowledge meaningfully. I want to be the wise child. Where should I go so that God finds me? I should not look at God from my point of view but look with God to embrace God's point of view. And from God's point of view, I arise as God's instrument by which he or she investigates, Is God necessary? Would God exist even if God did not exist? I need to be honest about God.

[Light playing...]

It took me years to accept the truth. It is shocking but enlightening.

God doesn't have to be good.

This is the sum of all wisdom. Eternal life is understanding that God doesn't have to be good. Let us take this step by step and then I will suggest how to model this with advanced mathematics.

God is beyond conditions. Good is God within conditions. Life is the fact that God is good, that the slack within conditions is in harmony with the wholeness beyond conditions. But eternal life is understanding that God doesn't have to be good. Goodness is within conditions but God is beyond conditions. Ultimately, if we are to grow, we have to let go of our conditions, identify with the unconditional, and take up richer conditions.

[Helmet with wilted flowers]

Simply put, eternal life is eternal growth. Life is not fair, and it can't be fair if we are to grow.

In math, with the concept of entropy we can model the ambiguity as regards two different outlooks, justice and grace. Justice supposes a closed system but grace supposes an open system. Which kind of system do we live in? It's completely ambiguous. Do we live on a planet where everything is falling apart? Or on a planet which absorbs energy for life? Life demands justice but eternal life commands grace. The human will loves the perfect but God's will loves the imperfect. It is not fair, it is not good, it is most Godly.

I am telling you the bad news. Jesus said, at the end of his life, "I have much more to tell you but you cannot bear it now. But when the Spirit of Truth comes, it will tell you all things." That is exactly the mission of Math 4 Wisdom. We manifest the Spirit of Truth as a language, a science, a community of wondrous wisdom.

[Plants]

Plants: I want to live in the spirit of the Indefinite.

Mu: Do you believe in the minimization operator mu?

Plants: Axiomatically? As a schema? Which takes an f and returns a mu of f? I think so. Sure.

Mu: You have to believe in me because otherwise all you got is primitive recursive functions. Now, if I find my zero, then I can't promise you nothing. But if I don't then I will keep searching and I will take this function f that you belong to and I will give you a new function mu of f that is indefinite - definitely indefinite - definitely indefinite.

[Photos and videos of Brother David Ellison-Bey]

...link bottom up (cognitive frameworks) and top down (speculation about God's point of view) with real life (by challenging ourselves to reach out to others.

In this Spirit we reach out to understand others and to understand ourselves and how to apply ourselves. That is how I met Brother David Ellison-Bey in 1997 in Chicago. I was organizing good will exercises to address situations which trouble us when we want to follow the truth of the heart but that clashes with the truth of the world. Brother David was a kindred spirit as I sought to apply wisdom to peacemaking, social justice, cultural autonomy, community organizing, personal morality, engaging God, enriching the public domain, making a living, falling in love and personal growth. I will be making a series of Math 4 Wisdom videos and organizing related activity to address such practical subjects with wondrous wisdom. If we seek true wisdom, then we must care whether, what, how and why wisdom matters.

I am sad to say that Brother David died just a few weeks ago. Math 4 Wisdom is greatly inspired by his Moorish Cultural Workshop. He taught youth and adults hundreds of creative educational activities, including yoga, nutrition, cooking, etiquette, recycling, caring for dogs, cats and fish, collecting bugs, gardening, music, fashion, the literature of East and West, local, national and international politics, the racial caste system, the history and religion of the Moors, archiving and curating, hospitality, organizing meetings, managing money, earning money and accounting.

[Footage with Brother David]

Brother David's deepest value in life was compassion, and a question he pondered was, How to get people to know their real self?

[Antonio Jesus and Andrius Kulikauskas - deepest values and investigatory questions]

What do I see from the top of the mountain?

I see exercises in Math 4 Wisdom that you can do to absorb the impact of this episode

• For wisdom, ask yourself, what is your deepest value in life? What is a question that you don't know the answer to but wish to answer?
• For math 4 wisdom, figure out the meaning of all of these math symbols. You can find them in the Wikipedia articles on the topics I mentioned in the subtitles. And you can try to read and understand those Wikipedia articles so you can start to know everything!
• For deciding to support math 4 wisdom through Patreon, master the radical teaching by Jesus to give everything away, figure out what that would mean for you practically, model that mathematically to minimize anxiety, compare that with what I wrote in the paper "An Economy for Giving Everything Away" which I authored with Brother David Ellison-Bey, and research the impact that we had upon high school student Chris Messina, and how his invention changed the world, facilitating these social movements and many more.

I hear the truth of the fivesome for decision making, conceived as time or space!

"Every effect has had its cause, but not every cause has had its effect, so there is a critical point for deciding."

What have I decided to give away? All of my original work is always in the Public Domain, copyright free, for you to use and share in your own best judgement. This is the spirit of genius, the spirit of inspiration, the spirit of truth, the spirit of Math 4 Wisdom.

Do you know what? This is not the top of the mountain. I can see higher vantage points. This is just a local maximum. So there will be more episodes of Math 4 Wisdom! At least for one year and maybe more with your support.

I end this episode with thanks to all who bless Math 4 Wisdom.

I offer a prayer to God to recognize God's centrality in wisdom. My prayer is inspired by my elder, Brother David Ellison-Bey, and what I learned from him in his Moorish Cultural Workshop.

[Wrap up]

Before I pray, I want to reflect on what I learned from making this episode.

I have been thinking about the search for constancy, which gives rise to one, all, many.

Constancy is one of four conceptions of the nullsome, the division of everything into zero perspectives, which models God.

Search is relevant in the parable of the lost child, the pinnacle of wondrous wisdom.

Thinking about that, I realized that the searching takes place on the level of shared understanding, where child and parent both understand that there needs to be a search. So I want to investigate the connection between all four conceptions of nullsome and all four levels of understanding.

Also, the whole point of the minimization operator mu is to ground the indefinite, which is the original state of God.

I am curious to explore that with Math 4 Wisdom!

Why am I sitting on these steps?

When I was a child in California, about 1970, my mother wanted me to watch a television show, "Sesame Street", that she said would be very important. A man named Gordon sat on the steps and explained how we need to make an effort to get along with people of different races and different places. I am grateful for his impact on me, and also for the show's creative energy in educating.

I hope likewise to influence you and others with Math 4 Wisdom, perhaps graduate students or former graduate students or future graduate students, who are hungry for more.

[Prayer]

---

Target audience, please give a round of applause to math educators who are preparing you for upcoming episodes of Math 4 Wisdom.

This is an update on the Patreon supporter function. Input is: Zero supporters Output is: Zero euros. Zero dollars. Zero Saudi riyals.

Mu: Zero! Eureka! I found it!

Minimization operator mu has terminated. Target audience please stay tuned for a special preview of Foursome starring Yoneda Lemma.

---

Plants: I hear the truth of the fivesome for decision making conceived in space or time.

Fivesome: Every effect has had its cause but not every cause has had its effect so there is a critical point for deciding to preview Fivesome starring Sheffer Orthogonal Polynomials.

Math 4 Wisdom: Educators I Thank

• Timothy Gowers
• John Baez
• Frederic Schuller
• Tobias Osborne
• Daniel Chan
• Norman Wildberger
• Richard Borcherds
• Emily Riehl
• Richard Southwell
• Sean Carroll
• Tai Danae Bradley
• Xavier Viennot
• Dr Physics A
• Tom Leinster
• Pierre Albin
• Allen Hatcher
• Roger Penrose
• David Griffiths
• Anthony Zee
• Federico Ardila
• David Tong
• Saurabh Basu
• Luis Gregorio Dias
• Robert Harper
• Ravi Vakil
• Egbert Rijke

I submitted this video to the "Summer of Math Exposition". I did not place in the top 100. I did benefit from the attention of about two hundred viewers. Thank you!