• View
• Edit
• History
• Print

Andrius Kulikauskas: I am working on the paper below. I wish to give a talk at the Models of Consciousness Conference.

---

Modeling Introspected Contexts With Mutually Anticommuting Linear Complex Structures

Abstract

A linear complex structure Jₖ distinguishes the unitary group U(n) of matrix actions from the symmetric space O(2n)/U(n) of compatible contexts. I explore how Jₖ could model an introspected perspective, bridging an unconscious mind M₁ that knows answers personally, and a conscious mind M₂ that asks questions, contextually. Applying such mutually anticommuting structures J₁, J₂, ..., J₈ to O(16r), r∈N, yields a chain of Lie group embeddings, O(16r) ⊃ U(8r) ⊃ Sp(4r) ⊃ Sp(2r)×Sp(2r) ⊃ Sp(2r) ⊃ U(2r) ⊃ O(2r) ⊃ O(r)×O(r) ⊃ O(r), with period 8, manifesting Bott periodicity. J₁, J₂, ..., Jₖ generate a Clifford algebra. They could possibly model the division of the mental workspace into k perspectives which together define a mental context. For example, such a context could structure k=2 existential attitudes (free will, fate), k=3 modes of a learning cycle (take a stand, follow through, reflect), or k=4 levels of knowledge (whether, what, how, why). Conceivably, Unconscious M₁ adds a perspective, Conscious M₂ adds a perspective on a perspective, and Consciousness M₃ adds a perspective upon this perspective on a perspective, all modulo 8, inhabiting an eight-cycle of metaphysical contexts, imposing complex, quaternionic and split-biquaternionic structure, respectively.

Outline

Introduction

• Academic context for my colleagues. Modeling our inner realm as an inner realm.
• Historical context for idealists. Stopping and thinking.
• Evolutionary context for materialists. Modeling the known and the unknown.

Preliminaries

• Grappling with different manifestations of Bott periodicity.
  • Homotopy groups
  • Clifford algebras
  • Topological insulators
• Perspective and mental reflection.
• Mathematically modeling reflection.
• Clifford algebras of perspectives.
• Classical Lie groups.

Mathematical Model

• Lie group embeddings.
• Embracing knowledge of the knower.
• Discarding context leads to failure.

Philosophical Interpretation

• Clifford algebras as divisions of everything
• Three minds in human culture.
• Divisions of everything in human culture.

Discussion

• Implications for theories of consciousness.

Introduction

Academic context for my colleagues. Modeling our inner realm as an inner realm.

Mathematical models of consciousness generally assume that we observe and interact with phenomena situated in a preexisting logic, space and time. Inevitably, such assumptions have us conclude that an externally oriented framework is prevalent and inherent in all of our thinking. What is the alternative? We may attempt to model how our minds grapple with a mental blank state, bring sense to utter abstraction, unfold a metaphysics, derive consistency out of contradiction, and imagine a primordial spirit, prior to everything, including logic, space, time, thought and mathematics.

Bott periodicity is a wonder of mathematics, which describes the way holistic structures fit together, as if manifesting the symmetry inherent in mathematical thinking itself. Bott periodicity, both eightfold real and twofold complex, has found application in condensed matter physics, where it coordinates symmetries which are profound not just physically but metaphysically: whether time is reversible, whether holes are particles, and whether we can distinguish a mirror world from our own. Bott periodicity, as expressed in a cycle of Lie group embeddings, constructs compact symmetric spaces (all ten of them!) in terms of mutually anticommuting linear complex structures {$J_k, 1\leq k\leq 7$}, and also {$iJ_1$}, where all of these {$J_k$} are isomorphic! Repeated application of linear complex structure generates and cycles through all symmetric spaces, which can be interpreted to say, all possible contexts for experience.

My goal is to show that these Lie groups and their quotients, the symmetric spaces, are worthy of consideration in modeling how we experience our inner life, our most abstract introspections, our various levels of consciousness. I join in a tradition of philosophical mathematicians, including Grassmann, Clifford, Peirce, Whitehead, Spencer-Brown, Varela, Kauffman, Bohm, Hiley, Conte, Northrop, Lehar, Goertzel and Goldstein, who have sought transcendent meaning in logic and algebra, and more recently, Clifford algebra.

Mastering Bott periodicity or simply comprehending the literature requires an all-around understanding of algebraic topology, homotopy theory, differential geometry, differential topology, calculus of variations, Morse theory, functional analysis, operator theory, complex analysis, real analysis, category theory, homological algebra, algebraic geometry, projective geometry, symplectic geometry, ring theory, group theory, representation theory, symmetric spaces, Clifford algebras, Grassmannians, division algebras, superalgebras, quaternions, octonions, random matrices, quantum mechanics, topological insulators, Hamiltonians, spinors, differential equations, combinatorics and K-theory, which is to say, all of math except for number theory. I myself have a PhD in algebraic combinatorics and a very limited understanding of these many subjects.

For me, Bott periodicity is an Everest-Sagarmāthā-Qomolangma which I dream of climbing, yet not for its own sake, but to explore whether it mirrors an eight-cycle of conceptual structures, divisions of everything, which I have been documenting in my life of introspection. Thus I am presenting to my fellow climbers the routes which I believe can avail us the vistas I seek.

Lie theory, which brings together algebra and analysis, is needed for a deep understanding of the chain of Lie group embeddings, which I will focus on. For any natural number {$r>0$} we have:

{$$O(16r)\supset U(8r)\supset Sp(4r)\supset Sp(2r)\times Sp(2r)\supset Sp(2r)\supset U(2r) \supset O(2r)\supset O(r)\times O(r) \supset O(r)$$}

Lie groups are, on the one hand, multiplicative groups, and on the other hand, differentiable manifolds. Of central importance are the orthogonal group {$O(n)$}, unitary group {$U(n)$} and compact symplectic group {$Sp(n)$}. These are matrix groups and much of what is relevant can be comprehended with a decent knowledge of linear algebra, as taught in an engineering mathematics course, and as presented at Wikipedia.

In this exposition, I point out aspects of these mathematical structures which are relevant for modeling our introspected lives, as in defining a perspective, distinguishing direct and indirect experience of a perspective, and establishing eight mental contexts that carve up everything and organize it into {$k$} perspectives, {$0\leq k\leq 7$}. I will describe three levels of awareness as operations which develop contexts by adding a perspective, a perspective on a perspective, or a perspective upon a perspective on a perspective. I identify this third operation with consciousness.

Historical context for idealists. Stopping and thinking.

Scientists regularly declare that a new approach is needed to understand consciousness. And yet perhaps we have abandoned what is actually needed, namely, the traditional quest to achieve wisdom, understand why, know everything, discover absolute truth, interact with God, know oneself.

The inspiration for this paper arose from a personal journey dating back to the 1980s when, in the academic scientific community, the study of consciousness was considered taboo, but also notions of why, causality, meditation, animal emotions, plant communication, prayer, extraterrestrials, panpsychism. Introspection is widely considered unreliable. The scientific study of God is still a taboo, to this day, and absolute truth all the more so.

In 1982, when I was a freshman at the University of Chicago, we were encouraged to ask big questions, but discouraged to think they could have answers. All truth was relative, whereas I sought absolute truth. I wished to know everything but could I know anything? Complex concepts are defined in terms of simpler concepts. But how can we define the most basic concepts? I concluded that the simplest concepts must define themselves by their relationships with each other. I experienced these as perspectives which are related by shifts in perspective.

When professor Michael Gillespie asked us, What is happiness? I noted the three kinds of answers that my fellow students gave. For some, it was joy, as in eating ice cream. For others, it was contentment, as in reflecting upon that. And for others, it was the assurance, that it was real and right. Thus the question triggered this distinction of doing and thinking and being. How were these modes related? I think therefore I am, and likewise, I am therefore I do, I do therefore I think. There was a three-cycle of participation, of learning through embodiment, as with the scientific method. We take a stand - assert a hypothesis, follow through - conduct an experiment, and reflect upon the results.

I also noted an endless debate regarding free will and fate. From the perspective of free will, opposites coexist, as with good and bad, but from the perspective of fate, it is all the same. Rather than take sides, I observed that both perspectives were necessary for existence. We need to be able to inquire whether a chair exists or not, and we also need to be able to answer definitely, which is the case. My mind shifts readily from the lack of knowledge in the question to the knowledge given in the answer. But once I have the answer, I do not readily go back to that same question. My mind readily shifts from the perspective of free will to that of fate but not the other way around.

Another basic holistic structure consists of four levels of knowledge. Plato's Republic distinguishes between false opinion (knowing what, sensually) and true opinion (knowing how, functionally) and wisdom (knowing why, comprehensively). To these I added the opposite of wisdom, ignorance (knowing whether, blindly). I collected examples of how great thinkers structured perspectives holistically into conceptual frameworks.

In this way, I intuited that decision making, whether in space or time, requires five perspectives: Every effect has had its cause, but not every cause had had its effects, and so there is a critical point for deciding. Morality organizes six perspectives. Absolutely, we can take a stand, follow through and reflect. Relatively, we can experience moral challenges: I take a stand, but do I follow through? I follow through, but do I reflect? I reflect, but do I take a stand?

In 1989, as an independent student at Vilnius University in Soviet-occupied Lithuania. My advisor, Rolandas Pavilionis, told me about Algirdas Greimas's semiotic square, based on Aristotle's logical square, which I interpreted as a division of everything into seven perspectives. Adding an eighth perspective, such as "All are known and all are unknown", would force the system to be empty, so that the divisions come to their end. There could be a division of everything into no perspectives, as with a state of contradiction, as with God beyond system. And there could be a division of everything into one perspective, namely, everything itself.

Pavilionis also suggested that I read Immanuel Kant's Critique of Pure Reason. My analysis of his Transcendental Deduction brought me to think that there were operations that were acting on these divisions of everything. Thus issues of existence (appealing to two perspectives) when we had full consciousness of them, became issues of decision making (appealing to five perspectives), upon documenting that consciousness as well. Consciousness could be understood as adding three perspectives, or more precisely, a perspective upon a perspective on a perspective. This could be denoted with equations such as 2+3=5 which would be understood modulo 8 as they took place on an eight-cycle of divisions of everything. The operations +1, +2, +3 added one or two or three perspectives, respectively, and thus there were 24 equations to introspect and document. This was quite daunting and so I worked further, for decades, on various aspects of the big picture, developing a private language which I hoped could be ultimately recognized as a unviersal language, true for all people and all systems.

In 2011, my sister Rima gave me "Thinking, Fast and Slow" by Daniel Kahneman about his work with Amos Tversky in experimental psychology. Their experiments distinguished between a fast thinking System 1 and a slow thinking System 2. Gradually, I understood this as a distinction between an intuitive, unconscious mind {$M_1$} which instinctively knows the answer and a talkative, conscious mind {$M_2$} which does not know but asks questions. {$M_1$} expresses a neural network of 100 billion neurons, and {$M_2$} expresses a conceptual model with 100 thousand concepts. {$M_1$} speaks with our emotions to let {$M_2$} know that its model is inadequate. {$M_2$} updates its model and imposes it cognitively upon {$M_1$}. I thought of {$M_1$} as a first level of reflection and {$M_2$} as the second level, reflection upon reflection, also know as awareness. I surmised from my earlier work that there should be a mind {$M_3$} which keeps {$M_2$} from rushing, makes sure the model brings peace to {$M_1$}, balances these two minds so they say the same thing in their own different ways, and finally chooses which of these minds we should act with in the matter at hand.

From 2014 to 2018, including a stint as a philosopher at VGTU, I gave 40 academic presentations of these and related ideas. Some found them interesting but none found them compelling. Thus, in 2022, I founded Math 4 Wisdom, a supportive investigatory community for absolute truth, to explore where these cognitive frameworks arise in the practice of advanced mathematics. I published a video how the Yoneda Embedding expresses four levels of knowledge: whether, what, how, why there is an arrow. I investigated the orthogonal Sheffer polynomials, which appear in solutions to Schroedinger's equation. Their fivefold classification can be identified with the five zones of a scattering problem: asymptotically free (Meixner), the arisal of a wave function (Charlier), a bound state (Laguerre), the collapse of a wave function (Hermite), and subsequent entanglement (Meixner-Pollaczek). These zones can be matched with the division of everything into five perspectives for decision making, as with space or time. I explored how divisions of everything could be modeled with chain complexes and exact sequences. Most boldly, I set my sights on making sense of Bott periodicity, whether it models the eight-cycle of divisions of everything.

I wonder about my own neurodiversity, what is peculiar about me that I fixate on these structures, which I believe we all must be familiar with, from when we first grow conscious, for we all seem able to learn, understand and apply a basic substrate of abstract outlooks. I wish to think most deeply and so I abstract away from all experience, shutting down {$M_1$}, and I let go of all conceptual language, unplugging {$M_2$}, considering what is left in that abstract void, the realm of {$M_3$}, the land of absolutes. Thus I stop and think, freeze my thinking, keep myself from wandering off, and instead stay within the same mental context, balancing on the same tipping point, looking out in all directions. Like a blind person feeling the walls of a cave, I stay within my chamber but discover its exits and how they are related. I know myself.

Mythologically, I imagine, I am a wombling, thinking the original, primordial thoughts, contemplating the limits of imagination itself, without experience, without preconceptions, avoiding the spectacles of the sunlit world. We may sleep in the womb, but what do we dream, if not the chambers of our minds, which are so familar, we don't stop to think of them? I urge us to recall that dream, return to the womb, go back to the cave.

Evolutionary context for materialists. Modeling the known and the unknown.

An alternative myth, offering an evolutionary perspective, which scientists may find agreeable, is that systems devote resources to prepare for and model the known but also to prepare for and model the unknown. There is a tendency towards devoting more and more resources to model what is not known. Thus there is evolution towards ever more prediction and abstraction. Evidence for the embodied mind is also evidence for a disembodying mind.

A single-celled paramecium knows whether. It engages the world directly by way of its receptors for light and chemicals. But a butterfly has a mind {$M_1$} that knows what. It lives in a world of flowers, that is, a neural representation of the world in terms of sensorial images which it pays attention to. A mouse has a mind {$M_1$} but also a mind {$M_2$} that knows how, that inhabits an abstract world of indexical and causal relationships. As neuroscientist Michael Graziano has noted, a mouse has awareness, in that it utilizes a model of attention. It can model its own attention, and thus be itself aware, and likewise model a cat's attention, and thus be aware of whether or not the cat is attending to the mouse.

Humans and perhaps the great apes can moreover have a mind {$M_3$} that knows why, that has consciousness, that lives in a world of choices. We can choose what we wish to be aware of. Birute Galdikas has noted how orangutang males go off to live alone, as if they were Zen Buddhists, and how they can choose to ignore people or not. We humans can choose with {$M_3$} to "step in" with {$M_1$} and immerse ourselves in a subjective experience, or to "step out" with {$M_2$} and consider objectively what is going on, what others are experiencing.

At the height of consciousness, our world reduces to addressing abstract choices, adjusting our attitude, recognizing our mental context, distinguishing the parameters by which we govern ourselves, choosing and pulling our internal switches, by which we roll down one or another slope of our tipping point, whereforth our subordinate minds {$M_1$} and {$M_2$} proceed on auto-pilot. All of this comes to a head abstractly, within our inner voids, where we choose to be more or less relaxed, proactive or reactive, generous or critical, tentative or decisive, and in every case, we choose to set up the abstract context from which we will continue when we return to this state of consciousness. For we have consciousness fleetingly, at critical tipping points, like a flickering flame. We experience consciousness by losing it, hopefully, by investing it as we lose it, returning us to the top of a ridge, where we awaken to our consciousness anew. Otherwise, we are brought to consciousness infrequently, accidentally, not of our own will but through the chance of life and love.

To know whether, what, how, why is also to not know but ask whether? what? how? why? The materialist emphasizes the answers, most prominently whether, and dismisses why. The idealist emphasizes the questions, most prominently why, and dismisses whether. Evolution favors the idealists. Our resources shift from answer to question as we evolve from whether to why.

The brain has a map of the body. Likewise, it may have an atlas of the mind. That atlas may have maps of our mental workspace, how its resources get carved up into {$k$} perspectives, with {$0\leq k\leq 7$}. Each map describes a tipping point, in abstract space, a context for choice amongst {$k$} perspectives. The atlas furthermore organizes how our minds move from one tipping point to another, adding one or two or three perspectives, as I have proposed. All three minds act on these maps and thus act in synchrony. Ultimately, in our inner depths, we are abstract beings, walking along the corners of a cube or the faces of an octohedron, or less poetically, walking along an eight-cycle, as all systems tend to, potentially, actually, necessarily. Such is my hypothesis in this work-in-progress.

I now share my thoughts on how Bott periodicity, and in particular, the chain of Lie group embeddings, can model this atlas of the mind.

Preliminaries

Grappling with different manifestations of Bott periodicity.

Eightfold real and twofold complex Bott periodicity manifest themselves, respectively, in homotopy groups of {$O(\infty)$} and {$U(\infty)$}, as well as {$GL(\mathbb{R},n)$} and {$GL(\mathbb{C},n)$}, the loop spaces of {$BO(\infty)$} and {$BU(\infty)$}, the reduced suspensions of {$BO(\infty)$} and {$BU(\infty)$}, the {$KO$}-theory and {$K$}-theory of spheres, the matrix representations of real and complex Clifford algebras, and the real and complex spin representations. Bott periodicity is found in the previously mentioned Lie group embedding as well as the classifications of symmetric spaces, super division algebras and topological insulators. It can be understood as multiplication by the canonical octonionic line bundle over {$\mathbb{O}\mathbb{P}^1$}.

Which of these manifestations are most relevant, informative, helpful, intuitive, comprehendible, learnable in modeling our inner life? The most compact and straightforward way for me to present my intuitions is to share the intellectual adventure by which I gained them.

Homotopy groups

I stumbled upon Bott periodicity in 2016. I was returning to math and physics with the ambition to show how my conceptual language would enable me to make sense of how it all unfolds. I made a map showing how 60 branches of math, used to classify journal articles, depended on each other. At the center was Lie theory, bringing together algebra and analysis. Amazingly and intriguingly, there were only four infinite families of classical Lie groups and algebras. The compact groups were the orthogonal group of even and odd dimensions, {$O(2n)$} and {$O(2n+1)$}, the unitary group {$U(n)$} and the compact symplectic group {$Sp(n)$}. These preserved lengths as expressed in real numbers, complex numbers and quaternions, thus were central to geometry. As I learned more, I came across John Baez's articles and the Wikipedia article on Bott periodicity.

In 1957, Raoul Bott astounded the world with his revelation that, for large enough {$n$}, the homotopy groups exhibit a twofold periodicity {$\pi_k(U(n))=\pi_{k+2}(U(n))$} and eightfold periodicity {$\pi_k(O(n))=\pi_{k+8}(O(n))$}. This meant that the homotopy groups of {$n$}-spheres likewise but unexpectedly settled into an eightfold periodicity when {$n$} was large enough. For when {$n$} is small compared to {$k$} the homotopy groups exhibit no intelligible pattern but rather chaotic noise. Whereas Bott proved that the noise clears away when {$n$} is large.

I was struck at once with the question whether this may manifest the eight-cycle of divisions of everything. Bott's theorem was beyond my comprehension, except to appreciate that homotopy groups of spheres {$\pi_k(S^n)$} consist of equivalence classes of continuous maps from {$k$}-spheres to {$n$}-spheres. It was believable that such maps could be modeling perspectives. That was the only clue for quite some time.

Bott's periodicity theorem yielded the patterns {$\pi_k(U(n))=0,\mathbb{Z}$} for {$k=0,1$} and {$\pi_k(O(n))=\mathbb{Z}_2, \mathbb{Z}_2, 0, \mathbb{Z}, 0, 0, 0, \mathbb{Z}$} for {$k=0,\dots ,7$}. Even if I could understand these patterns, they were simply indicators of deeper structure that I would need to flesh out for my purposes. I studied the first chapters of Hatcher's Algebraic Topology on homotopy theory and homology. But ultimately I would need to understand {$K$}-theory and {$KO$}-theory, the study of complex and real vector bundles over a topological space or scheme. Arguably, this was the most powerful and difficult branch of mathematics, developed by Grothendieck, Serre, Atiyah and Hirzebruch. I looked for an easier, more direct and relevant approach.

Clifford algebras

The classification of the representations of Clifford algebras offers another, much more concrete way to understand Bott periodicity. Clifford algebras generalize the construction of numbers, starting with the real numbers {$Cl(R)_{0,0}$}, the complex numbers {$Cl(R)_{0,1}$}, the quaternions {$Cl(R)_{0,2}$}, and then proceeding higher, each time adding a generator {$e_k$} and doubling the number of dimensions. Here I am assuming that {$e_k^2=-1$} for all {$k$}. There is also a rule that {$e_ie_j=-e_je_i$}. Putting this together, we can identify the basis elements of {$Cl(R)_{0,k}$} with the {$2^k$} terms in the product {$(1+e_1)(1+e_2)\dots (1+e_k)$}. The two extremes of this product are the identity {$1$}, when we choose no generators, and the pseudoscalar {$e_1e_2\cdots e_k$}, when we choose all generators. The pseudoscalar provides insight into the nature of the Clifford algebra {$Cl(R)_{0,k}$}. The square of the pseudoscalar has a fourfold periodicity {$-1,-1,1,1,-1,-1,1,1,\dots$}

{$e_1e_1=-1, (e_1e_2)(e_1e_2)=-1,$}

{$(e_1e_2e_3)(e_1e_2e_3)=1, (e_1e_2e_3e_4)(e_1e_2e_3e_4)=1,$}

{$(e_1e_2e_3e_4e_5)(e_1e_2e_3e_4e_5)=-1, (e_1e_2e_3e_4e_5e_6)(e_1e_2e_3e_4e_5e_6)=-1$}

and so on. Later on, we will need the fact that {$(e_\alpha e_\beta)^2=-1$} whereas {$(e_\alpha e_\beta e_\gamma)^2=1$} when {$\alpha, \beta, \gamma$} are all different.

Combinatorially, in each case we are swapping {$1+2+3+\dots +(k-1)$} generators and then squaring {$k$} generators. This contributes a sign {$(-1)^{\frac{k(k+1)}{2}}$}. We can observe how the exponent grows. It starts out odd {$1$}, then we add an even number {$2$} and the sum is odd {$3$}. Then we add an odd number {$3$} and the sum {$6$} is now even. We add an even number {$4$} and the sum stays even {$10$}. We add an odd number {$5$} and it switches to odd {$15$}. Thus it grows: odd, odd, even, even, odd, odd, even, even... This is elementary and concrete but what can it mean? We need to look deeper.

A real Clifford algebra {$Cl(R)_{p,q}$} has {$p$} generators which square to {$+1$} and {$q$} generators which square to {$-1$}. For example, the quaternions {$\mathbb{H}$} are isomorphic to {$Cl(R)_{0,2}$} which has two generators, {$e_1,e_2$} and four basis elements, {$1, e_1, e_2, e_1e_2$}, which can be interpreted as {$1, i, j, k$}. We also have {$Cl(R)_{1,0}\cong\mathbb{R}\oplus\mathbb{R}$} and {$Cl(R)_{2,0}\cong M_2(\mathbb{R})$}, the {$2\times 2$} real matrices. Starting with these initial Clifford algebras, we can apply recursion relations {$Cl_{p+2,q}\cong Cl_{q,p}\otimes Cl_{2,0}\cong Cl_{q,p}\otimes M_2(\mathbb{R})$} and {$Cl_{p,q+2}\cong Cl_{q,p}\otimes Cl_{0,2}\cong Cl_{q,p}\otimes \mathbb{H}$} to identify the larger Clifford algebras with matrix algebras. These relations intertwine an internal, algebraic progression {$\mathbb{R},\mathbb{C},\mathbb{H}$}, with an external, matrix progression {$\mathbb{R},\mathbb{R}\oplus\mathbb{R},M_2(\mathbb{R})$}.

Starting with {$Cl(R)_{0,0}\cong\mathbb{R}$}, and adding generators that square to {$-1$}, we get:

{$Cl(R)_{0,0}\cong\mathbb{R}$}, {$Cl(R)_{0,1}\cong\mathbb{C}$}, {$Cl(R)_{0,2}\cong\mathbb{H}$}, {$Cl(R)_{0,3}\cong\mathbb{H}\oplus\mathbb{H}$}, {$Cl(R)_{0,4}\cong M_2(\mathbb{H})$}, {$Cl(R)_{0,5}\cong M_4(\mathbb{C})$}, {$Cl(R)_{0,6}\cong M_8(\mathbb{R})$}, {$Cl(R)_{0,7}\cong M_8(\mathbb{R})\oplus M_8(\mathbb{R})$}, {$Cl(R)_{0,8}\cong M_{16}(\mathbb{R})$}

Whereas if we add generators that square to {$+1$}, we get:

{$Cl(R)_{0,0}\cong\mathbb{R}$}, {$Cl(R)_{1,0}\cong\mathbb{R}\oplus\mathbb{R}$}, {$Cl(R)_{2,0}\cong M_2(\mathbb{R})$}, {$Cl(R)_{3,0}\cong M_2(\mathbb{C})$}, {$Cl(R)_{4,0}\cong M_2(\mathbb{H})$}, {$Cl(R)_{5,0}\cong M_2(\mathbb{H})\oplus M_2(\mathbb{H})$}, {$Cl(R)_{6,0}\cong M_4(\mathbb{H})$}, {$Cl(R)_{7,0}\cong M_8(\mathbb{C})$}, {$Cl(R)_{8,0}\cong M_{16}(\mathbb{R})$}

Which way is relevant for modeling our inner life? Does a generator model a perspective? Does a perspective square to {$-1$} or {$+1$}? And what would that mean?

Either way, we end up at {$M_{16}(\mathbb{R})$}. By the Wedderburn-Artin theorem, it is Morita equivalent to {$\mathbb{R}$}, which we started from. Morita equivalence means that from the point of view of category theory, looking from the outside, they are indistinguishable in terms of their representation theory. This is the collapse which expresses Bott periodicity and could also express the collapse of the eight-cycle of the divisions of everything.

However, this Morita equivalence is not informative enough. Every matrix algebra in the real numbers is Morita equivalent. {$M_8(\mathbb{R})$} is likewise Morita equivalent to {$\mathbb{R}$} and to {$M_{16}(\mathbb{R})$}. Similarly for the matrices of complex numbers and of quaternions.

The eightfold periodicity of Clifford algebra representations was known earlier than Bott's result on homotopy groups. In 1963, Bott, Atiyah and Shapiro connected the two results with their paper "Clifford modules". Thus we can refer to Clifford algebras to understand the pattern {$\mathbb{Z}_2, \mathbb{Z}_2, 0, \mathbb{Z}, 0, 0, 0, \mathbb{Z}$} Dale Husemoller's "Fibre Bundles" provides a detailed exposition. Each Clifford algebra which is a matrix algebra has a single irreducible representation. We can identify {$m$} copies with the number {$m$}, and identify all possibilities with the group {$\mathbb{Z}$} of integers. Whereas each Clifford algebra which is a direct sum of two matrix algebras has two irreducible representations and we can identify all of its representations with the group {$\mathbb{Z}\oplus\mathbb{Z}$}. Now consider the representation of the complex numbers {$Cl_{0,1}\cong\mathbb{C}$} in terms of {$2\times 2$} matrices.

{$$a+be_1 \rightarrow a+bi \rightarrow \begin{pmatrix}a & -b \\ b & a\end{pmatrix}$$}

Now consider what happens if we ignore the generator {$e_1$}, for example, setting {$b=0$}. Then this is a representation of the real numbers {$a$}. Indeed, it is a reducible representation, being two copies of the irreducible {$1\times 1$} representation of the real numbers.

{$$a\rightarrow \begin{pmatrix}a & 0 \\ 0 & a\end{pmatrix}$$}

We can think of this as defining a map from {$\mathbb{Z}$} to {$\mathbb{Z}$}, where the irreducible representation of the complex numbers gets mapped to these 2 copies of the irreducible representation of the real numbers. Quotienting out by this map leaves {$\mathbb{Z}/2\mathbb{Z}\cong\mathbb{Z}_2$}, which matches the homotopy group {$\mathbb{Z}_2$} in the pattern.

Perspective and Mental Reflection

The difference between "stepping in", direct experience, and "stepping out", reflected experience.

Perspective is their compatibility in a specific instance.

Felt meaning.

Twin opposites vs. marked opposites

Historical context (me stepping in) vs. evolutionary context (me stepping out).

I love you - consciousness holds both together as compatible.

7+3=2 Self-standing opposite - "good without bad". You model that in two ways. As everything (without a perspective) and everything (with a perspective). Combining the two (unreflected and reflected) gives the twosome. Self-standing disarms the self-standing opposite, provides context allowing for contradiction. Twosome: contradiction vs. noncontradiction.

Complex structure models this consciousness of compatibility - it doesn't matter which is the unreflected or the reflected.

Imposing complex structure is quaternionic? Linear (twin opposites) vs. antilinear (unmarked input and marked output because of conjugation). Throwing out the prejudicial convention of marked opposites.

Mathematically Modeling Reflection

Different ways of modeling mental reflection.

Linear complex structure distinguishes the tangent space from the manifold

The manifold describe locations and the tangent space describes orientations.

The manifold can be understood in terms of group actions. The tangent space is understood in terms of the Lie algebra commutator. When the commutator with J is zero, then elements commute with J.

J is both a rotation (location) and a skew-symmetric matrix (orientation). J belongs to the orthogonal group, unitary group and symplectic group.

Involution

Inverse

Transpose

Defining orthogonal, unitary, compact symplectic groups.

Negative

Note that for {$s\in S\cong O(2m)/U(m)$} we have {$s^{-1}=s^T=-s$}

{$MM^T=I$}

Reflection of a sphere

Inversion of a sphere. Antipodal map.

• If a sphere is centered at the origin, then an inversion symmetry maps any point {$\vec{p}$} to {$-\vec{p}$}. This is the antipodal map.

Inversion of a point. Symmetric space.

More generally, an inversion symmetry (a point inversion) about p is defined as x* = 2p − x.

Symmetric space. For each point p of M, there exists an isometry of M fixing p and acting on the tangent space {$T_pM$} as minus the identity. Globally, this is an isometry such that locally, at p, all tangent vectors end up mapped to tangent vectors in the opposite direction. Thus, locally at point p, there is an inversion of its tangent space.

Complex conjugate (and transpose)

{$z=x+yi\Rightarrow \bar{z}=x-yi$}

Quaternionic conjugate (and transpose)

{$q=x_0 + x_1i + x_2j + x_3k \Rightarrow \bar{q}=x_0 - x_1i - x_2j - x_3k$}

Antilinear operator

An antilinear map {$j$} on a vector space {$V$} over {$\mathbb{C}$} is an additive map for which {$j(\lambda v)=\bar{\lambda}j(v)$} for all {$\lambda\in\mathbb{C}$}, {$v\in V$}. Consider, for example, the quaternion {$j$}, for which {$ji=-ij$}, and the complex scalar {$\lambda = a+bi$}.

{$$j(\lambda v)=j(a+bi)v=(aj+bji)v=(aj-bij)v=(a-bi)j(v)=\bar{\lambda}j(v)$$}

In this context, where {$j^2=-1$}, {$j$} is called a quaternionic structure.

Eigenvalue of -1

Consider a matrix {$M$} such that {$M^2=+1$}. Then {$M^2-1=0$}, thus {$(M+1)(M-1)=0$} and {$(M+1)(M-1)v=0$} for all {$v\in V$}, where {$M$} acts on {$V$}. This means that its possible eigenvalues are +1 and -1 and if {$M\neq I$} and {$M\neq -I$}, then {$M$} has at least one eigenvalue of either. Consequently, if {$M$} acts on a real vector space {$V$}, then {$V=V_+\oplus V_-$} where {$Mv=v$} for {$v\in V_+$} and {$Mv=-v$} for {$v\in V_-$}. Thus {$M$} is rotation-free. It acts on {$V_+$} as the identity and it acts on {$V_-$} as a reflection. {$V_+$} and {$V_-$} are reducing subspaces, a spectral decomposition.

Isometry

Time reversal

Charge conjugation

Parity

Skew-symmetric matrix {$AA^T=-I$}

Skew-Hermitian matrix {$AA^H=-I$}

Quaternionic skew-Hermitian matrix {$AA^\dagger=-I$}?

Category theory

Linear complex structure as the context for rotation

We can distinguish context (symmetric space) and action (unitary group). Rotoreflection is associated with symmetric space and rotation with action. Reflection takes us from action to context.

Clifford Algebras of Perspectives

I make good use of the paper, lean on it and flesh out parts so it would be more understandable for those like us with a simpler mathematical background, linear algebra.

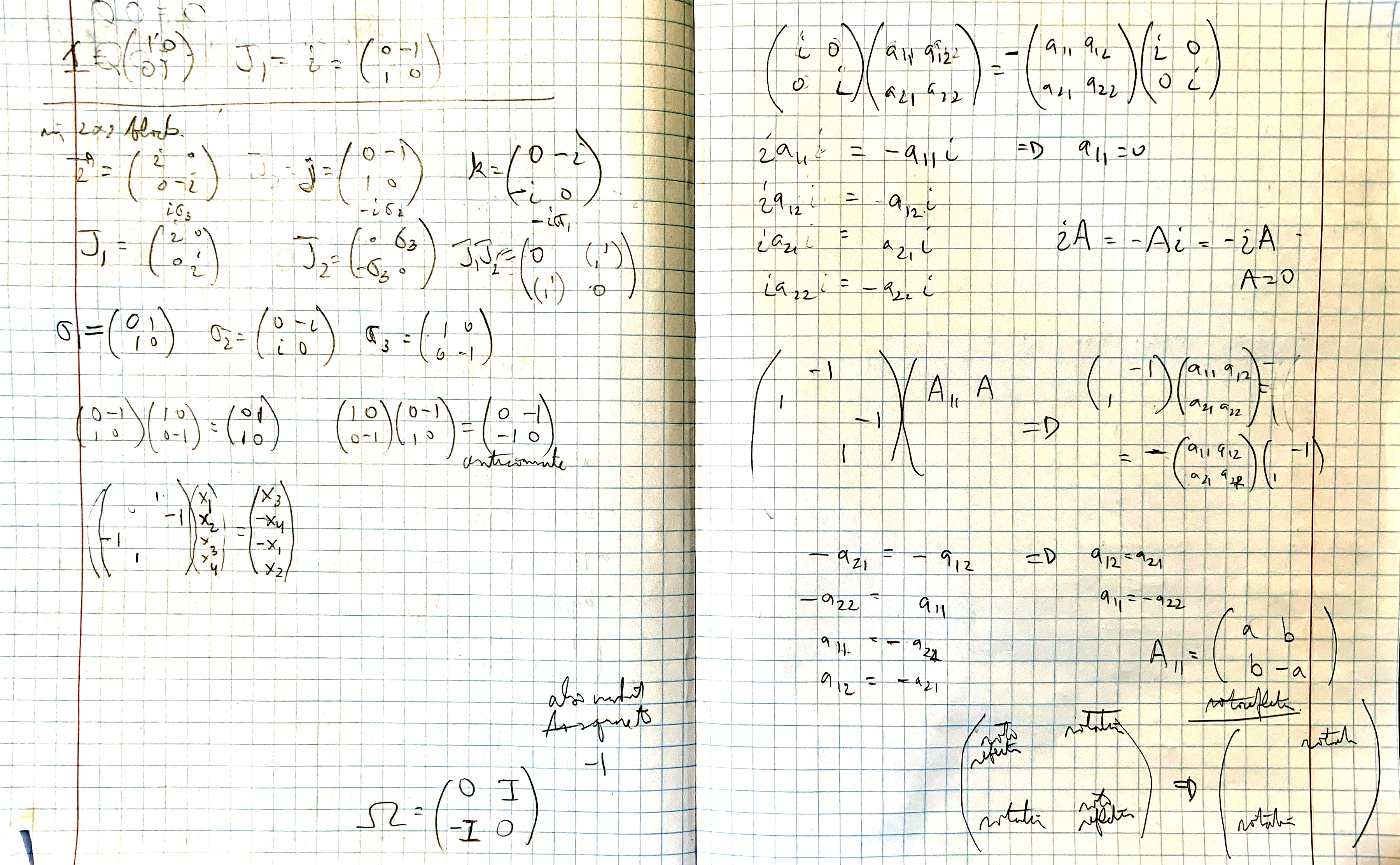
Linear complex structure {$J_k$} is an orthogonal matrix such that {$J_k^2=-1$}.

It can be diagonalized as {$AJA^{-1}$} where {$A$} is an orthogonal matrix and {$J$} is a block diagonal matrix of the form

{$$J=\textrm{diag}\begin{bmatrix}\begin{pmatrix} 0 & -1 \\ 1 & 0 \end{pmatrix}, \dots, \begin{pmatrix} 0 & -1 \\ 1 & 0 \end{pmatrix}\end{bmatrix}$$}

Namely:

{$$J=\begin{pmatrix}0 & -1 & & & \\ 1 & 0 & & 0 & \\ & & \ddots & & \\ & 0 & & 0 & -1 \\ & & & 1 & 0 \end{pmatrix}$$}

Given an orthogonal matrix {$O$}, it either commutes {$OJ=JO$} or anticommutes {$OJ=-JO$} with {$J$}. This is to say, {$OJO^{–1}=J$} or {$OJO^{-1}=-J$}. Conjugation of {$J$} yields {$J$} or its conjugate {$-J$}.

There can be two different linear complex structures {$J_1=A_1JA_1^{-1}$} and {$J_2=A_2JA_2^{-1}$}. This means that the matrix {$J$} can be understood in two different contexts, implemented with regard to two different bases, given by {$A_1$} and {$A_2$}. Indeed, we can have {$J_1$} and {$J_2$} be anticommuting, {$J_1J_2=-J_2J_1$}, which emphasizes that {$J_1\neq J_2$}.

We are interested in mutually anticommuting linear complex structures {$J_1,J_2,\dots, J_k$}. They are the generators for a real Clifford algebra {$Cl_{0,k}$} which has {$2^k$} basis elements. In particular:

{$Cl_{0,0}\cong\mathbb{R}$} has one basis element {$1$}.

{$Cl_{0,1}\cong\mathbb{C}$} has two basis elements {$1,J_1$} where we may identify {$J_1\leftrightarrow i$}.

{$Cl_{0,2}\cong\mathbb{H}$} has four basis elements {$1,J_1,J_2,J_1J_2$} where we may identify {$J_1\leftrightarrow i$}, {$J_2\leftrightarrow j$}, {$J_1J_2\leftrightarrow k$}.

{$Cl_{0,3}\cong\mathbb{H}\oplus\mathbb{H}$} has eight basis elements {$1,J_1,J_2,J_3,J_1J_2,J_1J_3,J_2J_3,J_1J_2J_3$} where we may identify {$1\leftrightarrow (1,1)$}, {$J_1\leftrightarrow (i,i)$}, {$J_2\leftrightarrow (j,j)$}, {$J_1J_2\leftrightarrow (k,k)$} and {$J_3\leftrightarrow (k,-k)$}, {$J_1J_3\leftrightarrow (-j,j)$}, {$J_2J_3\leftrightarrow (i,-i)$}, {$J_1J_2J_3\leftrightarrow (-1,1)$}.

Clifford algebras keep doubling, growing ... write them out ... whereas the Lie groups will be shrinking. They manifest Bott periodicity.

In analyzing Clifford algebras, it is important to note the properties of the products of various sizes, notably {$J_a$}, {$J_aJ_b$}, {$J_aJ_bJ_c$}. What are their squares? We calculate them using the basic facts that {$J_x^2=-1$} and {$J_xJ_y=-J_yJ_x$} when {$x\neq y$}. Then {$(J_a)(J_a)=-I$} and {$(J_aJ_b)(J_aJ_b)=-I$} whereas {$(J_aJ_bJ_c)(J_aJ_bJ_c)=+I$}. A consequence of the latter fact is that the matrix {$M=J_aJ_bJ_c$} acts on the vector space {$V$} by breaking it up {$V=V_+\oplus V_-$} where {$Mv=v$} for {$v\in V_+$} and {$Mv=-v$} for {$v\in V_-$}.

Classical Lie Groups

Compare the norms for the real number vector space {$\mathbb{R}^4$}, complex number vector space {$\mathbb{C}^2$} and quaternion vector space {$\mathbb{H}$}. They are all the same as quadruples {$a,b,c,d$}. Simply, there are different multiplicative rules defining them as algebras.

Complex numbers treat the personal and the contextual as equally valid. Real numbers emphasize one and discard the other. Quaternions accept both yet introduce that same prejudice a step deeper, in context.

The purpose of numbers x is to encode weights {$x_{ij}$} implications (i implies j). Proper numbers encode them in two directions: (i implies j) and (not j implies not i). Thus proper numbers are complex numbers. Real numbers are not proper numbers because they express only half of the information. Quaternions are abbreviated in that they express double the information.

Complex numbers are most plainly written as {$2\times 2$} matrices, in which case we clearly see that there are two imaginary numbers, twins, where the antidiagonal elements can have 1 above -1 or the other way around. The imaginary numbers are then defined by the fact that {$m^T=-m$}. This relates a twin opposite (given by the transpose) with a marked opposite. So it eliminates the distinction of marked and unmarked opposites.

{$iJ$} expresses the imaginary number in two ways, internally to mathematical structure as {$i$} and externally upon mathematical structure as {$J$}. This squares to {$1$}. Compare this to the quaternions, whose generators all square to {$-1$}.

Natural bases are orthonormal, with basis elements orthogonal and of unit length. There are three notions of length: real, complex, quaternionic. In the real case, we have to distinguish between odd and even. Even is natural and applies to all cases. Odd is unnatural, terminates periodicity and I think generates chaos.

The unitary group, based on the complex numbers, is most natural. The reason is how symmetry pairs up orthonormality equations. When basis elements are different, their relation gets written twice, and so we divide that by two. One complex equation yields two real equations, and so we get one real equation per entry. When basis elements are the same, the we get only one real equation, which is real because we are multiplying complex entries with their complex conjugates. Thus we get one real equation per entry. But if we have the orthogonal or symplectic group, then the diagonal elements count differently than the off diagonal elements. This has to do with the usage of "same" and "different" as regards "to" and "from". "From A to B" and "From B to A" count as two different entries, whereas "From A to A" counts as one entry.

Consider how choice frameworks relate to the widgets at the end of Lie algebra Dynkin diagrams, how they relate going forwards and backwards, how that compares to the relationship between identity and pseudoscalar for Clifford algebras.

Orthogonal group elements can be understood as actions but also as inputs and as outputs.

In the orthogonal group, both rotations and reflections make sense. A rotation is a pair of reflections, which implement a change of basis. In the unitary group, only rotations make sense. In the symplectic group, ...

Symplectic form {$J=\begin{pmatrix}0 & -I_n\\ I_n & 0\end{pmatrix}$} manifests the complex number {$i$}, when {$n=1$}, and can also be thought as the diagonal {$2\times 2$} block matrix. All three reflections coincide: {$J^{-1}=-J=J^T$}. For a symplectic matrix {$M$} we have {$M^TJM=J$}, thus {$JMJ^{-1}=(M^T)^{-1}$}.

Wikipedia: O(2n) is the maximal compact subgroup of GL(2n, R), and U(n) is the maximal compact subgroup of both GL(n, C) and Sp(2n). Thus the intersection O(2n) ∩ GL(n, C) or O(2n) ∩ Sp(2n) is the maximal compact subgroup of both of these, so U(n). From this perspective, what is unexpected is the intersection GL(n, C) ∩ Sp(2n) = U(n).

Wikipedia: Decomposing a Hermitian form into its real and imaginary parts: the real part is symmetric (orthogonal), and the imaginary part is skew-symmetric (symplectic)—and these are related by the complex structure (which is the compatibility). On an almost Kähler manifold, one can write this decomposition as h = g + iω, where h is the Hermitian form, g is the Riemannian metric, i is the almost complex structure, and ω is the almost symplectic structure.

For complex matrices there is the decomposition {$M=UT$} into unitary and upper triangular. The upper triangular matrices are homotopy equivalent to the diagonal matrices and to the identity. So the general linear group over the complex numbers is homotopy equivalent to the unitary group.

Rotations and reflections

• A rotation is a pair of reflections.
• A pair of reflections is distinguished by a rotation of one reflection into the other.
• A rotoreflection is a reflection times a rotation. But the rotation is a pair of reflections. So can the rotoreflection's reflection be chosen so as to undo one of the reflections in the rotation, and leave the remaining reflection?
• All of this shows that bases define context, and rotations relate contexts. Are any two contexts, any two bases related by a rotation? I think yes, if we don't care about the orientation of the basis elements, but no, in that we may need to reflect one of them.

Dimensional analysis

• {$O(n)$} has {$\frac{1}{2}n^2-\frac{1}{2}n = \frac{n(n-1)}{2}$} real dimensions. This is {$n^2-(\frac{n(n-1)}{2}+n)$}.
• {$U(n)$} has {$n^2$} real dimensions. This is {$2n^2-(2\frac{n(n-1)}{2}+n)$}.
• {$Sp(n)$} has {$2n^2+n=n(2n+1)$} real dimensions. This is {$4n^2 - (4\frac{n(n-1)}{2}+n)$}.

The dimension is calculated by calculating the number of independent equations, then subtracting from the total number of variables (matrix entries times field dimension), to get the number of free variables.

In each case, the diagonals manifest a different self-relation, which is the reason for the discrepancies, and the wobbling behind the periodicity.

Unitary is the most regular. Note the Dynkin diagrams.

• {$A_n$} as with {$U(n+1)$}: {$3,2,1$}
• {$B_n$} as with {$O(2n+1)$}: {$3,2,1,0,-1,-2,-3$}
• {$C_n$} as with {$Sp(n)$}: {$3,2,1=-1,-2,-3$}
• {$D_n$} as with {$O(2n)$}: {$3,2,1,-1,-2,-3$}

Mathematical Model

Lie Group Embeddings

{$\begin{matrix} \mathbf{Lie\; group} & \mathbf{real\; dimensions} \\ U(2r) & 4r^2 \\ U(r)\oplus U(r) & 2r^2 \\ U(r) & r^2 \\ \end{matrix}$}

Complex Bott Periodicity

• Compare {$iJ_1$} with {$J_1J_2J_3$}. They both square to {$+1$}.

{$\begin{matrix} \mathbf{Lie\; group} & \mathbf{real\; dimensions} \\ O(16r) & 128r^2-8r \\ U(8r) & 64r^2 \\ Sp(4r) & 32r^2 + 4r \\ Sp(2r)\times Sp(2r) & 16r^2 + 4r\\ Sp(2r) & 8r^2+2r \\ U(2r) & 4r^2\\ O(2r) & 2r^2-r\\ O(r)\times O(r) & r^2-r\\ O(r) & \frac{1}{2}r^2-\frac{1}{2}r \end{matrix}$}

Off-kilter will show how minds are off-kilter, requiring three minds and eight mental states.

Note that {$O(16r)\nsupseteq U(8r) \nsupseteq O(8r)\times O(8r)$}

real dimension of {$O(8r)\times O(8r)$} is {$64r^2-4r$}

The dimension of a quotient (a symmetric space) has the same quadratic power as the group in the denominator.

Note that with {$J_7$} there must be unitary matrices which do not commute with {$J_7$} because otherwise we would go from {$O(2r)$} to {$U(r)$}.

The choice of {$J_k$} is given by the symmetric space {$G_k/G_{k+1}$} and {$G_k$} is the subgroup of {$G_0\equiv O(16r)$} that commutes with {$J_1,J_2,\dots J_k$}. Similarly, the choice of {$iJ_k$} is given by the symmetric space {$H_k/H_{k+1}$} where {$H_0\equiv U(2r), H_1\equiv U(r)\times U(r), H_2\equiv U(r)$}.

Each symmetric space {$G_i/G_{i+1}$} is naturally embedded (as a totally geodesic submanifold) in {$G_{i-1}/G_i$}. For {$G_i/G_{i+1}$} parametrizes the set of geodesics {$\gamma=J_i\textrm{exp}^{\pi J_i^{-1}J_{i+1}t}=J_i\cos\pi t+J_{i+1}\sin\pi t$} where {$J_i=\gamma (0)$} and {$J_{i+1}=\gamma(1/2)$} and {$-J_i=\gamma (1)$}.

The classification of symmetric spaces is given by these quotients and shows how fundamental, remarkable and special this chain is.

The set of choices for {$J_k$} is parametrized by {$G_k/H_k$} where {$J_k$} commutes with each element of {$H_k$}. But {$J$} itself belongs to {$H_k$}, I think, for all {$k$}, and I imagine {$J_k=DJD^{-1}$} as well. The parametrization is of the contexts {$D$}, the bases, whereby we have {$J_k=DJD^{-1}$}.

But what does this parametrization mean? How does it relate to the elements of {$G_k$} that {$J_k$} anticommutes with?

Rooting Out Reflective Knowledge Leads to Failure

William Kingdon Clifford. "It is wrong always, everywhere, and for anyone to believe anything on insufficient evidence."

The orthogonal group models all experience, what is known. What is true, which goes hand in hand with what is not true, what is false. Negation serves and functions as reflection.

Apply the linear complex structure {$J_1$} to distinguish the known from the unknown, the true from the false.

(What does it mean if, in four dimensions, we have two reflections?) Reorganizing the dimensions to pair eigenvalues and get rotations.

Active inference distinguishes what is not the organism from what is the organism. Thus distinguishes the conscious from the unconscious.

Apply {$J_2$} to distinguish the known known from the known unknown, which is to say, distinguish the knower. This yields an anti-linear operator.

Apply {$J_3$} to distinguish the known known known from the known known unknown. Then the operator {$J_1J_2J_3$}, which squares to {$+1$}, splits the vector space into subspaces with eigenvalues {$+1$} (nonreflective) and {$-1$} (reflective).

{$J_1, J_2, J_3$} function as a three-cycle on {$V_-$}, and in the opposite direction, on {$V_+$}.

Apply {$J_4$} to distinguish the known known known known from the known known known unknown. But at this point we have that {$J_3J_4$} is an isometry (a reflection) between unreflective {$V_+$} and reflective {$V_-$}, thus relating them.

The transformation {$J_3J_4J_5$} splits the space in two subspaces and on one of them {$J_3J_4=J_5$}. But that is true for every {$J_3J_4J_k$}.

This yields {$Sp(2)\subset Sp(2)\times Sp(2)$} which consists of the identifications of the unreflective and the reflective. The points in the diagonal {$Sp(2)$} are these identifications. How do these identifications evolve further? and are they maintained?

Applying {$J_5$}, {$J_6$}, {$J_7$} further splits the space yielding {$W_+$}, {$X_+$}, {$Y_+$} as we go along.

Note that {$J_3J_4J_5$} splits {$V$} into two spaces {$S_+$} where {$J_3J_4J_5v=v$} and {$S_-$} where {$J_3J_4J_5=-v$}. Then on {$S_+$} we have {$J_5=J_4J_3$} and on {$S_-$} we have {$J_5=J_3J_4$}. Thus we get a reversal of the shift between the two shifts of the foursome. But the same is true of any subsequent operator.

We have isometries {$W_-=J_2W_+$}, {$X_-=J_1X_+$}.

Then we have the isometry {$J_7J_8$} such that {$Y_-=J_7J_8Y_+$}.

Consider how adding more linear complex structures takes us down from {$O(2)$} to {$O(1)\times O(1)$} rather than {$U(1)$}. Note that {$SO(2)\cong U(1)$}.

The collapse arises because to proceed further we need to have the two subspaces {$O(n_1)$} and {$O(n_2)$} be the same size. Not just the same size, but they have to be exactly the same, both identical to the diagonal element.

But then the argument is that there is no distinction between the two spaces, what is known and what is not known. In the symplectic case we also have this issue but it is removed because it is on the level of knowledge. Note also that what was distinctly different and opposite has, through the symmetric space, become encoded in the same way, represented in the same way. So we are shifting through different kinds of opposites.

In the case of {$U(n_1)$} and {$U(n_2)$} and the diagonal we have the case of Jesus (the diagonal) equating God and human.

Consider how after we have laid down eight linear complex structures it is as if we had not laid down any and they no longer have an effect. In what sense is the system "empty"? In what sense is it true that "all are known and all are known" for what is left? In what sense is there a problem with {$G_7/G_8$}?

These symmetric spaces are Grassmannians of various flavors. They are ways of choosing various {$k$}-dimensional subspaces of various {$n$}-dimensional spaces. Philosophical Interpretation

Clifford Algebras as Divisions of Everything

Nullsome and onesome

Twosome

Threesome

Foursome

Fivesome

Sixsome

Sevensome

Three Minds in Human Culture

Neural network, enmeshed with the world, conveying and summarizing what is, what is known, what functions as answers. Conceptual language, apart from the world, expressing what is not, what is unknown, in the form of slots, variables, words, concepts, which function as questions.

• Logical square: Knowing Mind vs. Not-Knowing Mind
• Three minds express What, How, Why from the idealist point of view. Whether is God. Why functions like a break, allowing How to shift to What upon release, at which point Why becomes Whether.
• System 1 and System 2
• Preconscious processing and Conscious processing
• Right Hemisphere and Left Hemisphere
• Jung. Anima and animus.
• Yin and yang.
• Freud. Id, Superego, Ego.
• Polanyi: Tacit and Explicit
• Peirce: Firstness, Secondness, Thirdness.
• Plato: Subjects, Warriors, Rulers.
• Hegel: Thesis, Antithesis, Synthesis.
• Irrational and Rational.
• Perspective vs. Perspective on Perspective.
• First, Second, Third Person.
• Beautiful, good, true.
• Compare with a transistor.
• Traditional Understandings of Female and Male Preferences. Two stereotypic genders, two roles in dialogue, in traditional understanding, as pillars essential for robust dialogue. Building on those pillars as the basis for gender diversity. Full variety of nonstereotypic genders can be constructed based on their logical relationships.
• Neural network AI. Fast speed: Run-time inference. Dropping a pin ball and it cascades through. Medium speed: Attention/Modulation. Dopamine as an amplifier or diminisher. Sliding window of doubt that sets the neuromodulatory tone (or attitude or context in which the fast speed is received.) Lets you pop out of your local minimum. Slower scale: Structural topological, the actual connectivities, adding or removing connections.

Clifford

• Receiving evidence. “It is wrong always, everywhere, and for anyone to believe anything on insufficient evidence.”
• Forming beliefs. “It is wrong always, everywhere, and for anyone to ignore evidence that is relevant to his beliefs, or to dismiss relevant evidence in a facile way.”
• Governing how we form, hold, revise, relinquish beliefs over time. [If someone violates such a diachronic obligation by “purposely avoiding the reading of books and the company of men who call in question” his presuppositions, Clifford warns, then “the life of that man is one long sin against mankind”]

Divisions of Everything in Human Culture

Gestalt psychology?

Discussion

Implications for theories of consciousness

Gives a very clear definition of consciousness which allows for a wide variety of implementations. Much to offer to scientists and scholars pursuing distinct research programs.

Biology

• Ant colony. Ways of figuring things out: not centrally organized (divergent), centrally organized (convergent), and relating the two.
• Bioelectricity and proteins and DNA.
• Mitchell - free agent hierarchy

Neurology

• Evoke consciousness wherever there are two hemispheres. Baby, puppy, octopus.

Artificial intelligence

• Raudys hierarchy of statistical classifiers for neural networks

Global workspace

• There exists a map of the mind which neurology could find.
• The map could be perhaps in the basal ganglia.
• The hemispheres exist

Information integration theory

• There is only one way that k perspectives can assemble.
• Bott periodicity runs through all symmetric spaces. So this seems unavoidable, irresistible.
• Random matrix ensembles.
• Seems to encode the symmetry inherent in mathematics itself, the way that notation is interpreted, the ways that matrices can fold up.
• All systems are the same. The complexity is latent and it is just how much is manifest. Teleological.

Active inference

• The gap between the world and the organism can be understood as the gap between the unconscious and the conscious. For the unconscious is enmeshed in the world and the conscious is not. Sensation takes the form of the emotions by which the unconscious communicates to the conscious the insufficiency of the conceptual language. Action takes the form of cognition by which the Conscious imposes the updated conceptual language upon the Unconscious. Consciousness

Panpsychism

• Look for eight-cycles and Bott periodicity as a sign of consciousness.
• Cohl Furey and particle physics.
• Krebs cycle of metabolism in all cells.
• Octosulfur and the origin of life.

Theological

• Genesis. For God, a day of creation is a division of everything.
• Genesis. You will be like God rather than you will be God.
• God and love.
• Jesus.
• Three kinds of knowledge.

Philosophers

• Gives a ground for introspection.

Wokeism

• Universe is woke. Throws out prejudicial conventions.
• But are there ways of putting prejudices in contexts?

Morality

• {$iJ_1$} is relevant for connecting to people and to God.
• But the three-cycle {$J_1J_2J_3$} likewise plays that role, squares to {$+1$}.

Additional Topics

Homotopy Theory

CPT Symmetry

References

Sattin, D.; Magnani, F.G.; Bartesaghi, L.; Caputo, M.; Fittipaldo, A.V.; Cacciatore, M.; Picozzi, M.; Leonardi, M. Theoretical Models of Consciousness: A Scoping Review. Brain Sci. 2021, 11, 535. https://doi.org/10.3390/brainsci11050535

M Stone, CK Chiu, A Roy. Symmetries, dimensions and topological insulators: the mechanism behind the face of the Bott clock. Journal of Physics A: Mathematical and Theoretical, 2010. iopscience.iop.org https://arxiv.org/abs/1005.3213

Friedman, D.A., Søvik, E. The ant colony as a test for scientific theories of consciousness. Synthese 198, 1457–1480 (2021). https://doi.org/10.1007/s11229-019-02130-y

Henry D. Potter, Kevin J. Mitchell. Naturalising Agent Causation. Entropy 2022, 24, 472.

https://plato.stanford.edu/entries/ethics-belief/ Andrew Chignell. The Ethics of Belief. Stanford Encyclopedia of Philosophy.

https://people.brandeis.edu/~teuber/Clifford_ethics.pdf William K. Clifford. The Ethics of Belief. Contemporary Review, 1877.

Kauffman, L. H. (1987). "The fundamental structures of human reflexion": Comment. Journal of Social & Biological Structures, 10(2), 189–192. https://doi.org/10.1016/0140-1750(87)90006-6

V. A. Lefebvre. Algebra of Conscience. 2nd ed. Springer. 2001.

Elio Conte. A Clifford algebraic analysis gives mathematical explanation of quantization of quantum theory and delineates a model of quantum reality in which information, primitive cognition entities and a principle of existence are intrinsically represented ab initio*. World Journal of Neuroscience, 2013, 3, 157-170. https://www.scirp.org/pdf/WJNS_2013071910551255.pdf

Elio Conte, Ferda Kaleagasioglu, Rich Norman. Algebraic Quantum Theory of Consciousness. Aracne editrice. 2018. https://www.researchgate.net/publication/328879758_ALGEBRAIC_QUANTUM_THEORY_OF_CONSCIOUSNESS

Elio Conte. On the Logical Origins of Quantum Mechanics Demonstrated By Using Clifford Algebra: A Proof that Quantum Interference Arises in a Clifford Algebraic Formulation of Quantum Mechanics. Electronic Journal of Theoretical Physics. May 2011, Vol. 8 Issue 25, p109-126. 18p.

Koehler, G. Q-consciousness: Where is the flow? Nonlinear Dyn. Psychol. Life Sci. 2011, 15, 335–357.

Khrennikov A (2015) Quantum-like modeling of cognition. Frontiers of Physics. 3:77. doi: 10.3389/fphy.2015.00077

Louis H. Kauffman. The Mathematics of Charles Sanders Peirce. Cybernetics & Human Knowing, Vol.8, no.1–2, 2001, pp. 79-110.

Andrius Kulikauskas. Time and Space as Representations of Decision-Making. Presented at "Space and Time: An Interdisciplinary Approach", September 29-30, 2017 in Vilnius, Lithuania https://www.math4wisdom.com/wiki/Research/20170929TimeSpaceDecisionMaking

Tyler Goldstein. Sentient Singularity Theory. https://www.sentientsingularity.com

Michaele Suisse, Peter Cameron. Aims and Intention from Mindful Mathematics: The Encompassing Physicality of Geometric Clifford Algebra. 2017.

Lehar S. (2003b) "Gestalt Isomorphism and the Primacy of the Subjective Conscious Experience: A Gestalt Bubble Model". The Behavioral and Brain Sciences 26(4), 375-444. http://slehar.com/wwwRel/webstuff/bubw3/bubw3.html

Steven Lehar. Clifford Algebra: A Visual Introduction. https://slehar.wordpress.com/2014/03/18/clifford-algebra-a-visual-introduction/

Steven Lehar. The Boundaries of Human Knowledge: A Phenomenological Epistemology or Waking Up in a Strange Place. http://slehar.com/wwwRel/webstuff/book2/Boundaries.pdf

https://www.youtube.com/channel/UCj1M0P2I8zLLKlVUHb8xf2w/videos

Ben Goertzel. On the Algebraic Structure of Consciousness. Dynamical Psychology. 1996. https://www.goertzel.org/dynapsyc/1996/consalg.html

Ben Goertzel. Ons: An Algebraic Foundation for Being and Time, Explaining the Emergence of Clifford Algebra Structure. December 1997. (Rough Draft, Not for Distribution) https://www.goertzel.org/papers/OnsAlgebra.html

Ben Goertzel, Onar Aam, Tony Smith, Kent Palmer. Ons Algebra: The Emergence of Quaternionic, Octonionic and Clifford Algebra Structure From Laws of Multiboundary Form (Rough Draft, not for distribution) https://www.goertzel.org/papers/Multi.html

Ben Goertzel. Ons: a theory of truly elementary particles, explaining the emergence of structure from void in physics and psychology (Rough Draft, for comments only) November 1996.

Frank Dodd (Tony) Smith, Jr. - 2015 LHC 2015-16 and E8 Physics. https://vixra.org/abs/1508.0157

Basil Hiley. Quantum theory, the Implicate Order and Consciousness. Interview with Richard Bright (Editor: Interalia Magazine). Published in With Consciousness in Mind (Part 3) – November 2015. https://www.interaliamag.org/wp-content/uploads/2015/11/Basil-Hiley-Quantum-theory-the-Implicate-Order-and-Consciousness.pdf

B. J. Hiley. Process, Distinction, Groupoids and Clifford Algebras: an Alternative View of the Quantum Formalism. https://arxiv.org/pdf/1211.2107

Matti Pitkänen. Mathematical Aspects of Consciousness Theory

• An interesting special aspect of 8-dimensional Clifford algebra with Minkowski signature isthat it allows an octonionic representation of gamma matrices obtained as tensor products ofunit matrix 1 and 7-D gamma matrices γk and Pauli sigma matrices by replacing 1 and γk by octonions. This inspires the idea that it might be possible to end up with quantum TGD from purely number theoretical arguments. One can start from a local octonionic Clifford algebra in M8.